Solutions for theoretical problems in Physics January, 2002

In this page only the sketch of the solutions are published; in some cases only the final results. To achieve the maximum score in the competition more detailed solutions needed.

P. 3488. A solid, straight cylinder standing on its base is sliding down a slope of a given angle. The coefficient of friction is \(\displaystyle mu\). Which point of the cylinder's base can be considered as the work point of the pressing force exerted by the slope? (4 points)

Solution. The distance of the point from the symmetry axis of the cylinder is x=\(\displaystyle mu\)h/2.

P. 3489. A snowball of a mass of 150 g hits a window pane at a velocity of 20 m/s and stops within 0.01 s. Will the pane break if it can resist a maximum of 300 N pressing force at the site of the hit? (4 points)

Solution. The average of the pressing force is

\(\displaystyle F={\Delta(mv)\over t}={0.15\cdot20\over0.01}=300~\rm N.\)

The maximum force is larger than 300 N, therefore the pane will break.

P. 3490. Why is it that some helicopters need a tail-rotor and others do not? (3 points)

Solution. The helicopters having two rotors (rotating in opposite directions) need not any tail-rotor.

P. 3491. The pull force of an electric motor is 310 kN at a speed of 57.6 km/h. The supply voltage is 3.6 kV and there is an electric current of 230 A in the coils of the eight electric motors. Determine the efficiency of the motor. (3 points)

Solution. \(\displaystyle eta\)=F^.v/(8I^.U)=0.75.

P. 3492. What is the radius of a bubble that has formed from a detergent solution, and in which the density of air is one thousandth higher than the air surrounding it. (4 points)

Solution. \(\displaystyle R={4\alpha\over10^{-3}p_0}=1.3~\rm mm.\)

P. 3493. We make hydrogen gas undergo the ABCA thermal cycle shown in the figure. (p₁=2^.10⁵ Pa, V₁=2 m³, T_A=300 K.)

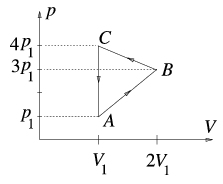
a) How much heat is transmitted to the gas during the different subprocesses?

b) What is the total amount of work performed on the gas? (4 points)

Solution. a) The internal energy \(\displaystyle E={5\over2}pV\) and the transmitted heat is \(\displaystyle Q=\Delta E-W={5\over2}\Delta(pV)+p\Delta V.\)

\(\displaystyle Q_{A\rightarrow B}={5\over2}\left(6p_1V_1-p_1V_1\right)+2p_1V_1=14{,}5p_1V_1=5800~\rm kJ,\)

\(\displaystyle Q_{B\rightarrow C}={5\over2}\left(4p_1V_1-6p_1V_1\right)+3{,}5p_1V_1=-8{,}5p_1V_1=-3400~\rm kJ.\)

\(\displaystyle Q_{C\rightarrow A}={5\over2}\left(p_1V_1-4p_1V_1\right)=-7{,}5p_1V_1=-3000~\rm kJ.\)

The total transmitted heat during the thermal cycle is

Q_total=Q_{A\(\displaystyle rightarrow\)B}+Q_{B\(\displaystyle rightarrow\)C}+Q_{C\(\displaystyle rightarrow\)A}=-600 kJ

. b)

The work performed on the gas is -Q_total=600 kJ.

P. 3494. What is the radius of the imaginary concentric sphere that divides the electrostatic field of a metal sphere of a radius of 20 cm and a charge of 8^.10^-7 C into two regions of identical energy? What is the total energy of the electric field of the metal sphere? (5 points)

Solution. The total energy of the electrostatic field of the metal sphere is

\(\displaystyle E=k{Q^2\over2R}=14{,}4~\rm mJ,\)

and one half of this energy is distributed in the region r>R^*=2R=40 cm.

P. 3495. The flat surface of a hemispherical glass lens is mat (n=1.5, R=4.8 cm). There is a point source of light on the axis of symmetry of the hemisphere. The incident light creates a bright spot on the mat surface. Where is the light source when the spot of light is the smallest? What is the diameter of the spot in this case? (5 points)

Solution. The diameter of the smallest spot is

\(\displaystyle r=r_{\rm min}={R\over n}=3.2~\rm cm,\)

and the distance between the light source and the centre of the hemisphere

\(\displaystyle d=R{n\over\sqrt{n^2-1}}=6.4~\rm cm.\)

P. 3496. \(\displaystyle lambda\)_min denotes the shortest wavelength of the light emitted by a hydrogen atom. Can this atom emit a photon of a wavelength of \(\displaystyle 2\lambda_{\min}\)? (4 points)

Solution. The hydrogen atom can not emit such a photon, because the equation

\(\displaystyle {1\over n^2}-{1\over m^2}={1\over2}\)

has not any integer solution.

P. 3497. We divert a string pendulum of a length of 1 meter until it is horizontal, then let it fall. What is the angular velocity of the acceleration vector of the body hanging at the end of the string when the acceleration vector is a) vertical, b) horizontal? (6 points)

Solution. The angular velocity of the acceleration vector is

\(\displaystyle \omega_a=3{1+2\tan^2\varphi\over1+4\tan^2\varphi}\cdot{2g\sin\varphi\over l},\)

where \(\displaystyle varphi\) is the angle of the string measured from the horizontal direction.

- At the beginning (\(\displaystyle varphi\)=0) the acceleration vector is vertical and its angular velocity is zero.

- At the angle \(\displaystyle varphi\)=35,3^o the acceleration vector is horizontal and its angular velocity is \(\displaystyle omega\)_a\(\displaystyle approx\)3,36 s^-1.

- At the deepest point of the path (\(\displaystyle varphi\)=90^o) the acceleration vector is vertical again and \(\displaystyle omega\)_a\(\displaystyle approx\)6,64 s^-1.