Problem A. 378. (September 2005)

A. 378. Does there exist a function $f:\mathbb{Q}\to\{-1,1\}$ such that f(x)=-f(y) whenever x and y are different rationals and xy=1 or x+y 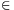$\in$ {0,1}?

(5 pont)

Deadline expired on October 17, 2005.

Solution. There exists such a function.

Consider the continued fraction form of the rational numbers. As is well-known, each rational x can be uniquely written as

$x=a_0+\frac1{a_1+\frac1{a_2+\dots+\frac1{a_n}}}$

where a₀ is an integer, a₁,...,a_n are positive integers and a_n>1.

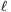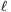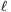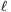For an arbitrary rational x, lett $\ell$ x be the number of divisions in the continued fraction form of x (the number n above). Then $\ell$ (x)=0, for inegers and $\ell$ (x)= $\ell$ (1/x)+1 for all 0<x<1.

Now define function f. Set

f(0)=-1; $f(x)=(-1)^{\ell(x)}$ for all x>0; $f(x)=-(-1)^{\ell(|x|)}$ , for all x<0.

By the definition of 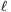$\ell$ (x) we have that for all positive rational x and positive integer k, 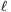$\ell$ (x+k)= 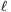$\ell$ (x) and f(x+k)=f(x).

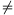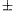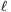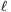Now prove f(1/x)=-f(x), if x $\ne$ 0, $\pm$ 1. Since f(-x)=-f(x) and f(-1/x)=-f(1/x), it can assumed that x>0. Moreover, the roles of x and 1/x are symmetric so we can assume 0<x<1. Then $\ell$ (x)= $\ell$ (1/x)+1, therefore f(1/x)=-f(x).

From the definition of f we know that f(x)=-f(y) for all x+y=0, except x=y=0. The final property which must be verified is that f(1-x)=-f(x) if $x\ne\frac12$ . By the symmetry assume $x>\frac12$ .

If x>1 then f(x)=f(x-1)=-f(1-x), done.

If x=1 then f(x)=f(1)=1 and f(1-x)=f(0)=-1, done.

The only remaining case is when $\frac12<x<1$ . then

$f(x)=-f \left(\frac1x\right)= -f\left(\frac1x-1\right)= -f\left(\frac{1-x}x\right)=$

$=f\left(\frac{x}{1-x}\right)= f\left(\frac{x}{1-x}+1\right)= f\left(\frac1{1-x}\right)= -f(1-x).$

Therefore, function f satisfies all the required properties.

Remark. It is easy to see that there exist exactly two solutions: f and -f.

Statistics:

23 students sent a solution.

5 points: Erdélyi Márton, Gyenizse Gergő, Hujter Bálint, Jankó Zsuzsanna, Kisfaludi-Bak Sándor, Kónya 495 Gábor, Molnár 999 András, Nagy 224 Csaba, Paulin Roland, Sümegi Károly, Szabó 108 Tamás, Ureczky Bálint.

4 points: Korándi Dániel, Radnai András, Szilágyi Dániel.

3 points: 2 students.

2 points: 3 students.

0 point: 3 students.

Problems in Mathematics of KöMaL, September 2005

23 students sent a solution.
5 points:	Erdélyi Márton, Gyenizse Gergő, Hujter Bálint, Jankó Zsuzsanna, Kisfaludi-Bak Sándor, Kónya 495 Gábor, Molnár 999 András, Nagy 224 Csaba, Paulin Roland, Sümegi Károly, Szabó 108 Tamás, Ureczky Bálint.
4 points:	Korándi Dániel, Radnai András, Szilágyi Dániel.
3 points:	2 students.
2 points:	3 students.
0 point:	3 students.

Already signed up?
New to KöMaL?