 Problem A. 476. (March 2009)
Problem A. 476. (March 2009)
A. 476. Let n 3 be an odd integer, and let A={0,1,...,n-1} denote the set of residual classes modulo n. Call a non-empty subset B
3 be an odd integer, and let A={0,1,...,n-1} denote the set of residual classes modulo n. Call a non-empty subset B A a Dutch set, if for every a
A a Dutch set, if for every a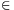 A and for every b
A and for every b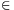 B at least one of b+a and b-a lies in B. Determine the smallest possible cardinality of a Dutch set in terms of n.
B at least one of b+a and b-a lies in B. Determine the smallest possible cardinality of a Dutch set in terms of n.
Proposed by: Gerhard Woeginger, Amsterdam
(5 pont)
Deadline expired on April 15, 2009.
Statistics:
13 students sent a solution. 5 points: Backhausz Tibor, Blázsik Zoltán, Bodor Bertalan, Éles András, Frankl Nóra, Nagy 235 János, Nagy 314 Dániel, Nagy 648 Donát, Tomon István, Weisz Ágoston, Wolosz János. 4 points: Tossenberger Anna, Varga 171 László.
Problems in Mathematics of KöMaL, March 2009
