Problem A. 574. (November 2012)

A. 574. Let n $\ge$ 2 and let $p(x)=x^n+a_{n-1}x^{n-1}+\ldots+a_1x+a_0$ be a polynomial with real coefficients. Prove that if for some positive integer k the polynomial (x-1)^k+1 divides p(x) then $\sum_{\ell=0}^{n-1} |a_\ell| > 1+\frac{2k^2}{n}$ .

CIIM 2012, Guanajuato, Mexico

(5 pont)

Deadline expired on December 10, 2012.

Solution. For convenience, define the leading coefficient a_n=1 also.

Lemma. For every polynomial q(y) with degree at most k, we have $\sum\limits_{\ell=0}^n a_\ell \, q(\ell) = 0$ .

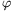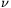
Proof. Let $\varphi$ ₀(y)=1 and let $\varphi_\nu(y)=y(y-1)\ldots(y-\nu+1)$ for $\nu=1,2,\ldots$ . By (x-1)^k|p(x), for 0 $\le$ $\nu$ $\le$ k we have

$\sum_{\ell=0}^n a_\ell \, \varphi_\nu(\ell) = f^{(\nu)}(1) = 0.$

Every polynomial with degree at most k can be repesented as a linear combination of the polynomials $\varphi_0(y),\ldots,\varphi_k(y)$ , so $q(y)=\sum\limits_{\nu=0}^k c_\nu \varphi_\nu(y)$ with some real numbers $c_0,\ldots,c_k$ . Then

$\sum_{\ell=0}^n a_\ell \, q(\ell) = \sum_{\ell=0}^n a_\ell \left( \sum_{\nu=0}^k c_\nu \, \varphi_\nu(\ell) \right) = \sum_{\nu=0}^k c_\nu \left( \sum_{\ell=0}^n a_\ell \, \varphi_\nu(\ell) \right) = 0.$

To prove the problem statement, let T_k be the kth Chebyshev-polynomial, and choose

$q(y) = T_k\left(\frac2{n-1}y-1\right).$

Then $q(0),\ldots,q(n-1)\in T_k\big([-1,1]\big)=[-1,1]$ , and

$q(n) = T_k\left(\frac{n+1}{n-1}\right) = \cosh \left( k\cdot \cosh^{-1} \frac{n+1}{n-1} \right) = \cosh \left( k\cdot \ln \left( \frac{n+1}{n-1} + \sqrt{\left(\frac{n+1}{n-1}\right)^2-1} \right) \right)$

$= \cosh \left( k\cdot \ln \frac{(\sqrt{n}+1)^2}{n-1} \right) = \cosh \left( k\cdot \ln \frac{1+\frac1{\sqrt{n}}}{1-\frac1{\sqrt{n}}} \right) > \cosh \frac{2k}{\sqrt{n}}.$

(In the last step we applied the inequality $\ln\frac{1+x}{1-x}>2x$ .)

By applying the lemma,

$\sum_{\ell=0}^{n-1} |a_\ell| \ge \sum_{\ell=0}^{n-1} a_\ell\big(-q(\ell)\big) = q(n) > \cosh \frac{2k}{\sqrt{n}} > 1+\frac{2k^2}{n}.$

Statistics:

3 students sent a solution.

5 points: Ioan Laurentiu Ploscaru.

1 point: 2 students.

Problems in Mathematics of KöMaL, November 2012

3 students sent a solution.
5 points:	Ioan Laurentiu Ploscaru.
1 point:	2 students.

Already signed up?
New to KöMaL?