 Problem B. 3870. (December 2005)
Problem B. 3870. (December 2005)
B. 3870. P is a point lying on an ellipse of foci F1 and F2, different from the endpoints of the major axis. Show that the value of
is independent of P.
(4 pont)
Deadline expired on January 16, 2006.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás: Legyen ,
és
, ekkor x+y=90o-z. Ha a szóban forgó mennyiséget u-val jelöljük, akkor, mivel u
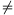 0,
0,
vagyis
Legyen PF2=a,PF2=b,F1F2=c, ekkor a+b csak az adott ellipszistől függ, vagyis
értéke is független P-től. Mivel sin z=cos (x+y)=cos xcos y-sin xsin y, kapjuk, hogy
Innen látszik, hogy értéke valóban csak magától az ellipszistől függ.
Statistics:
47 students sent a solution. 4 points: Almási 270 Gábor András, Blázsik Zoltán, Bock Lilla, Bogár 560 Péter, Csaba Ákos, Cseh Ágnes, Cserép Máté, Csizmadija Laura, Dányi Zsolt, Farkas Gergő, Fegyverneki Tamás, Herber Máté, Honner Balázs, Horváth 385 Vanda, Hülber Tímea, Kardos Kinga Gabriela, Komáromy Dani, Kornis Bence, Kovács 111 Péter, Kovács 129 Péter, Kozma Márton, Kunovszki Péter, Majoros Csilla, Nagy 235 János, Németh Kitti Noémi, Páldy Sándor, Pálovics Róbert, Pásztor Attila, Rábai András, Salát Zsófia, Sommer Dániel, Sümegi Károly, Szabó 108 Tamás, Szaller Dávid, Szalóki Dávid, Szentandrási István, Szűcs Gergely, Tallián György, Tomon István, Tossenberger Anna, Tóth 796 Balázs, Tóthmérész Lilla, Werner Miklós. 3 points: Peregi Tamás, Priksz Ildikó. Unfair, not evaluated: 2 solutionss.
Problems in Mathematics of KöMaL, December 2005
