Problem B. 4197. (September 2009)

B. 4197. Prove that if the sides of a triangle satisfy 2b²=a²+c², then the opposite angles satisfy 2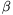cot =cot +cot .

(3 pont)

Deadline expired on October 12, 2009.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás. A koszinusz-tétel szerint \(\displaystyle b^2=a^2+c^2-2ac\cos\beta\), vagyis a feltétel ekvivalens azzal, hogy \(\displaystyle b^2=2ac\cos\beta\). Innen a szinusz-tétel alapján

\(\displaystyle 2\cos\beta=\frac{b}{a}\cdot\frac{b}{c}=\frac{\sin\beta}{\sin\alpha} \cdot\frac{\sin\beta}{\sin\gamma},\)

\(\displaystyle 2\text{ctg}\beta = \frac{\sin\beta}{\sin\alpha\cdot\sin\gamma}= \frac{\sin(\alpha+\gamma)}{\sin\alpha\cdot\sin\gamma}= \frac{\sin\alpha\cos\gamma+\sin\gamma\cos\alpha}{\sin\alpha\cdot\sin\gamma}= \text{ctg}\alpha + \text{ctg}\gamma.\)

Statistics:

89 students sent a solution.
3 points:	60 students.
2 points:	15 students.
1 point:	6 students.
0 point:	4 students.
Unfair, not evaluated:	4 solutionss.

Problems in Mathematics of KöMaL, September 2009