Problem K. 55. (November 2005)

K. 55. There are two milk bars in Cowton, and both of them have hot chocolate with frothed chocolate topping on their menus. Each milk bar serves in a cylindrical glass of the same height. (On serving, one half of the volume of the drink is liquid chocolate, and the other half is frothed chocolate.) In a short time, the froth turns into liquid chocolate of one quarter as much volume. In the Jolly Cowboy, chocolate is served in glasses of radius 6 cm, and sold for 12 Cowton cents a glass. In the Happy Cowgirl, the radius of the glasses is 5 cm, but they fill up the glass again when the froth of the first filling has settled. They charge 11 Cowton cents for a glass. In which milk bar is chocolate cheaper? [To obtain the volume of a cylinder, the area of its base is multiplied by its height.] (Based on a problem of the 24th József Öveges Memorial Competition)

(6 pont)

Deadline expired on December 12, 2005.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás: A Víg Tehénpásztorban a bögre térfogatának ${1\over2}+{1\over2}\cdot{1\over4}={5\over8}$ része a kapott kakaó mennyisége. A Jókedvű Csordásban a bögre térfogatának ${5\over8}+{1\over2}\cdot{3\over8}+{1\over2}\cdot{3\over8}\cdot{1\over4}={55\over64}$ része a kapott kakaó mennyisége. Legyen a bögrék magassága h cm! A Víg Tehénpásztorban a bögre térfogata köbcentiméterben mérve 36 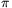$\pi$ ^.h, a kakaó mennyisége pedig $36\pi\cdot h\cdot{5\over8}={45\over2}\cdot\pi\cdot h$ , egy petákért $\left({45\over2}\cdot\pi\cdot h\right):12={15\over8}\cdot\pi\cdot h$ köbcenti jár. A Jókedvű Csordásban a bögre térfogata köbcentiméterben mérve 25 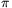$\pi$ ^.h, a kakaó mennyisége pedig $25\pi\cdot h\cdot{55\over64}={1375\over64}\cdot\pi\cdot h$ , egy petákért $\left({1375\over64}\cdot\pi\cdot h\right):11={125\over64}\cdot\pi\cdot h$ köbcenti jár. Mivel ${15\over8}={120\over64}<{125\over64}$ , ezért a Jókedvű Csordásban olcsóbb a kakaó.

Statistics:

231 students sent a solution.

6 points: 133 students.

5 points: 17 students.

4 points: 25 students.

3 points: 11 students.

2 points: 14 students.

1 point: 8 students.

0 point: 22 students.

Unfair, not evaluated: 1 solutions.

Problems in Mathematics of KöMaL, November 2005

231 students sent a solution.
6 points:	133 students.
5 points:	17 students.
4 points:	25 students.
3 points:	11 students.
2 points:	14 students.
1 point:	8 students.
0 point:	22 students.
Unfair, not evaluated:	1 solutions.

Already signed up?
New to KöMaL?