 Problem A. 380. (October 2005)
Problem A. 380. (October 2005)
A. 380. The convex n-sided polygon K lies in the interior of a unit square. Show that it is possible to select three vertices of the polygon that form a triangle of smaller area than units.
(5 pont)
Deadline expired on November 15, 2005.
Solution. We prove that there exist two adjacent sides of K which form a sufficiently small triangle.
K is convex and is a subset of the unit square. Therefore its perimeter p is at most 4.
Denote the length of the ith side of K by di and let the angle between the ith and (i+1)th sides be 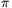 -
- i. Since
i. Since
there exists an index i for which .
Consider the triangle determnined by the ith and (i+1)th sides; its area is
Statistics:
15 students sent a solution. 5 points: Dücső Márton, Erdélyi Márton, Gyenizse Gergő, Hujter Bálint, Jankó Zsuzsanna, Kisfaludi-Bak Sándor, Kónya 495 Gábor, Nagy 224 Csaba, Paulin Roland, Tomon István. 4 points: Bogár 560 Péter. 1 point: 3 students. 0 point: 1 student.
Problems in Mathematics of KöMaL, October 2005
