Problem A. 468. (December 2008)

A. 468. We are given two triangles. Their side lengths are a,b,c and A,B,C respectively, the areas are t and T, respectively. Prove that -a²A²+a²B²+a²C²+b²A²-b²B²+b²C²+c²A²+c²B²-c²C² $\ge$ 16tT.

(5 pont)

Deadline expired on January 15, 2009.

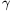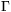Solution. Let $\gamma$ and $\Gamma$ the two angles opposite to sides c and C, respectively. From the cosine law we have a²+b²-c²=2abcos 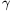$\gamma$ and A²+B²-C²=2ABsin 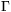$\Gamma$ ; the two areas are $t=\frac12ab\sin\gamma$ and $T=\frac12AB\sin\Gamma$ .

Substituing these quantities,

-a²A²+a²B²+a²C²+b²A²-b²B²+b²C²+c²A²+c²B²-c²C²-16tT=

=2a²B²+2A²b²-(a²+b²-c²)(A²+B²-C²)-16tT=

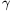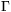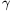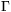=2a²B²+2A²b²-4abABcos $\gamma$ cos $\Gamma$ -4abABsin $\gamma$ sin $\Gamma$ =

=2(aB-Ab)²+4abAB(1-cos (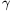 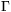$\gamma$ - $\Gamma$ )) $\ge$ 0.

Statistics:

12 students sent a solution.

5 points: Blázsik Zoltán, Bodor Bertalan, Éles András, Márkus Bence, Nagy 235 János, Nagy 648 Donát, Somogyi Ákos, Tomon István, Tossenberger Anna, Varga 171 László, Wolosz János.

4 points: Backhausz Tibor.

Problems in Mathematics of KöMaL, December 2008

12 students sent a solution.
5 points:	Blázsik Zoltán, Bodor Bertalan, Éles András, Márkus Bence, Nagy 235 János, Nagy 648 Donát, Somogyi Ákos, Tomon István, Tossenberger Anna, Varga 171 László, Wolosz János.
4 points:	Backhausz Tibor.

Already signed up?
New to KöMaL?