 Problem A. 517. (October 2010)
Problem A. 517. (October 2010)
A. 517. Let m 3 be a positive integer, and let
3 be a positive integer, and let  m(x) be the mth cyclotomic polynomial, and denote by
m(x) be the mth cyclotomic polynomial, and denote by 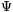 m(x) the polynomial with integer coefficients for which
m(x) the polynomial with integer coefficients for which . Prove that for every integer a, any prime divisor of the number
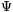 m(a) either divides m or is of the form mk
m(a) either divides m or is of the form mk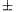 1.
1.
(5 pont)
Deadline expired on November 10, 2010.
Statistics:
5 students sent a solution. 5 points: Backhausz Tibor. 4 points: Ágoston Tamás, Nagy 235 János, Nagy 648 Donát. 3 points: 1 student.
Problems in Mathematics of KöMaL, October 2010
