Problem A. 546. (November 2011)

A. 546. Show that

$\frac{1}{\sin^2{\dfrac{\pi}{4k+2}}} + \frac{1}{\sin^2{\dfrac{3\pi}{4k+2}}} + \frac{1}{\sin^2{\dfrac{5\pi}{4k+2}}} + \ldots + \frac{1}{\sin^2{\dfrac{(2k-1)\pi}{4k+2}}} = 2k(k+1)$

for every positive integer k.

(5 pont)

Deadline expired on December 12, 2011.

Solution 1. Let U_n(x) be the nth Chebyshev-polynomial of the second kind, for which U_n(cos t)sin t=sin ((n+1)t). The roots of U_2k are the numbers $x_1=\cos\frac{\pi}{2k+1}=\sin\frac{(2k-1)\pi}{4k+2}$ , $x_2=\cos\frac{2\pi}{2k+1}=\sin\frac{(2k-3)\pi}{4k+2}$ , ..., $x_k=\cos\frac{k\pi}{2k+1}=\sin\frac{\pi}{4k+2}$ and their negatives, x_k+1=-x+1, x_k+2=-x₂, ..., x_2k=-x_k. On the left-hand side of the statement we have the sum of the squares of these numbers; the statement is equivalent with

$\sum_{j=1}^{2k} \frac1{x_j^2} = 4k(k+1).$

(1)

Lemma 1. U₀(x)=1, U₂(x)=4x²-1, and U_2k+2(x)+U_2k-2(x)=(4x²-2)U_2k(x) for k $\ge$ 1.

Proof. From U₀(cos t)sin t=sin t, we get U₀(x)=1. Similarly, from U₂(cos t)sin t=sin (3t)=(4cos²-1)sin t we get U₂(x)=4x²-1.

From the identity sin (a+b)+sin (a-b)=2sin acos b we obtain

U_2k+2(cos t)sin t+U_2k-2(cos t)sin t=

=sin ((2k+3)t)+sin ((2k-1)t)=2sin ((2k+1)t)cos (2t)=

=2U_2k(cos t)sin t^.(2cos²-1),

so U_2k+2(x)+U_2k-2(x)=(4x²-2)U_2k(x).

Lemma 2. Let $U_{2k}(x)=a_{k,0}+a_{k,1}x+\ldots+a_{k,2k}x^{2k}$ . Then a_k,0=(-1)^k, a_k,1=0 és a_k,2=(-1)^k+1^.2k(k+1).

Proof. Apply induction on k. If k=0 then U₀(x)=1, so a_0,0=1=(-1)^k, a_0,1=0, and a_0,2=0=-4k(k+1) If k=1 then U₀(x)=4x²-1, so a_1,0=-1=(-1)^k, a_1,1=0, and a_1,2=4=2k(k+1). Hence, the lemma holds for k=0 and k=1.

For the induction step assume the lemma for k and (k-1). By Lemma 1,

$a_{k+1,0}+a_{k+1,1}x+a_{k+1,2}x^2+\ldots=U_{2k+2}(x)= (4x^2-2)U_{2k}(x)-U_{2k-2}(x)=$

$= (4x^2-2)(a_{k,0}+a_{k,1}x+a_{k,2}x^2+\ldots) -(a_{k-1,0}+a_{k-1,1}x+a_{k-1,2}x^2+\ldots) =$

$= (4x^2-2)((-1)^k+(-1)^{k+1}\cdot 2k(k+1)x^2+\ldots) -((-1)^{k-1}+(-1)^k\cdot2k(k-1)x^2+\ldots).$

From this we read

a_k+1,0=-2^.(-1)^k-(-1)^k-1=(-1)^k+1,

a_k+1,1=0,

finally

a_k+1,2=4^.(-1)^k-2^.(-1)^k+1^.2k(k+1)-(-1)^k^.2k(k-1)=

=(-1)^k(4+4k(k+1)-2k(k-1))=(-1)^k+2^.2(k+1)(k+2).

The lemma is proved.

Now from the Viéte formulae we find

$\sum_{j=1}^{2k}\frac1{x_j^2} = \left(\sum_{j=1}^{2k}\frac1{x_j}\right)^2 - 2\sum_{1\le i<j\le 2k} \frac1{x_ix_j} = 0^2 -2\frac{a_{k,2}}{a_{k,0}} = -2\frac{(-1)^{k+1}\cdot 2k(k+1)}{(-1)^k} = 4k(k+1).$

Solution 2. Let 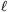$\varepsilon=\cos\dfrac\pi{4k+2}+i\sin\dfrac\pi{4k+2}$ , then $\ell$ egész számra $\sin\dfrac{\ell\pi}{4k+2} = \dfrac{\varepsilon^\ell-\varepsilon^{-\ell}}{2i}$ .

From the identity $a^n-b^n=(a-b)(a^{n-1}+a^{n-2}b+\ldots+b^{n-1})$ we have

$\frac{1}{\sin{\frac{(2j-1)\pi}{4k+2}}} = \frac{2i}{\varepsilon^{2j-1}-\varepsilon^{-(2j-1)}} =$

$= \frac{2i(\varepsilon^{2k(2j-1)}+\varepsilon^{(2k-2)(2j-1)} +\varepsilon^{(2k-4)(2j-1)}+\ldots+\varepsilon^{-2k(2j-1)})}{ (\varepsilon^{2k(2j-1)}+\varepsilon^{(2k-2)(2j-1)} +\ldots+\varepsilon^{-2k(2j-1)})(\varepsilon^{2j-1}-\varepsilon^{-(2j-1)})}=$

$= \frac{2i}{\varepsilon^{(2k+1)(2j-1)}-\varepsilon^{-(2k+1)(2j-1)}} \sum_{\ell=-k}^k \varepsilon^{2\ell(2j-1)} = \sum_{\ell=-k}^k \varepsilon^{2\ell(2j-1)},$

$\frac{1}{\sin^2{\frac{(2j-1)\pi}{4k+2}}} = \left(\sum_{\ell=-k}^k \varepsilon^{2\ell(2j-1)}\right)^2 = (2k+1) + \sum_{\ell=1}^{2k} \bigl(2k+1-\ell)(\varepsilon^{2\ell(2j-1)} + \varepsilon^{-2\ell(2j-1)}\bigr),$

$\sum_{j=1}^k \frac{1}{\sin^2{\frac{(2j-1)\pi}{4k+2}}} = \sum_{j=1}^k \left( (2k+1) +\sum_{\ell=1}^{2k} \bigl(2k+1-\ell)(\varepsilon^{2\ell(2j-1)} +\varepsilon^{-2\ell(2j-1)}\bigr) \right) =$

$= k(2k+1) + \sum_{\ell=1}^{2k} (2k+1-\ell) \sum_{j=1}^k \bigl(\varepsilon^{2\ell(2j-1)} + \varepsilon^{-2\ell(2j-1)}\bigr).$

Applying $a^n-b^n=(a-b)(a^{n-1}+a^{n-2}b+\ldots+b^{n-1})$ again,

$\sum_{j=1}^k \bigl(\varepsilon^{2\ell(2j-1)} + \varepsilon^{-2\ell(2j-1)}\bigr) = -\varepsilon^{-2\ell(2k+1)} + \varepsilon^{-2\ell}\sum_{j=-k}^k \varepsilon^{4\ell j} =$

$= -\varepsilon^{-2\ell(2k+1)} + \varepsilon^{-2\ell} \frac{\varepsilon^{2\ell(2k+1)}-\varepsilon^{-2\ell(2k+1)}}{ \varepsilon^{2\ell}-\varepsilon^{-2\ell}} = - (-1)^\ell + \varepsilon^{-2\ell} \frac{(-1)^\ell-(-1)^\ell}{\varepsilon^{2\ell}-\varepsilon^{-2\ell}} = (-1)^{\ell+1}.$

Therefore,

$\sum_{j=1}^k \frac{1}{\sin^2{\frac{(2j-1)\pi}{4k+2}}} = k(2k+1) - \sum_{\ell=1}^{2k} (2k+1-\ell) (-1)^\ell = 2k(k+1).$

Solution 3. We use U_2k again and prove (1). The polynomial can be factorized as $U_{2k}(x)=c\prod_{j=1}^{2k}(x-x_j)$ , where c is the leading coefficient. Then

$\frac{U_{2k}'(x)}{U_{2k}(x)} = \sum_{j=1}^{2k}\frac1{x-x_j},$

and

$\sum_{j=1}^{2k}\frac{1}{(x-x_j)^2} = \left(\sum_{j=1}^{2k}\frac{-1}{x-x_j}\right)'= \left(-\frac{U_{2k}'(x)}{U_{2k}(x)}\right)' = -\frac{U_{2k}''(x)}{U_{2k}(x)} +\left(\frac{U_{2k}'(x)}{U_{2k}(x)}\right)^2.$

Substituting x=0 we obtain

$\sum_{j=1}^{2k}\frac{1}{x_j^2} = -\frac{U_{2k}''(0)}{U_{2k}(0)}+\left(\frac{U_{2k}'(0)}{U_{2k}(0)}\right)^2.$

(2)

We compute the values U_2k(0), U_2k'(0), U_2k''(0) by differentiating

U_2k(cos t)sin t=sin ((2k+1)t)

(3a)

twice.

-U_2k'(cos t)sin²t+U_2k(cos t)cos t=(2k+1)cos ((2k+1)t),

(3b)

U_2k''(cos t)sin³t-3U_2k'(cos t)sin tcos t-U_2k(cos t)sin t=-(2k+1)²sin ((2k+1)t).

(3c)

By substituting t=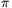 $\pi$ /2 in (3a--3c) we get U_2k(0)=(-1)^k and U_2k'(0)=0, furthermore

U_2k''(0)=(-1)^k-(-1)^k^.(2k+1)²=(-1)^k+1^.4k(k+1).

Plugging these into (2),

$\sum_{j=1}^{2k}\frac{1}{x_j^2} = -\frac{(-1)^{k+1}\cdot4k(k+1)}{(-1)^k}+0^2 = 4k(k+1).$

Statistics:

7 students sent a solution.

5 points: Ágoston Tamás, Gyarmati Máté, Janzer Olivér, Kovács 444 Áron, Mester Márton, Omer Cerrahoglu, Szabó 928 Attila.

Problems in Mathematics of KöMaL, November 2011

7 students sent a solution.
5 points:	Ágoston Tamás, Gyarmati Máté, Janzer Olivér, Kovács 444 Áron, Mester Márton, Omer Cerrahoglu, Szabó 928 Attila.

Already signed up?
New to KöMaL?