 Problem A. 552. (January 2012)
Problem A. 552. (January 2012)
A. 552. Prove that for an arbitrary sequence of nonnegative real numbers and
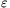 >0 there exist infinitely many positive integers n for which
>0 there exist infinitely many positive integers n for which .
(Schweitzer-competition, 2011)
(5 pont)
Deadline expired on February 10, 2012.
Solution. We prove by contradiction. Suppose that there is a sequence and
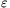 >0 for which
>0 for which
| (1) |
holds with finitely many exception. As a simple corollary of (1) we have
| 4an(1-an-1)>1; | (2) |
it follows that an>0 and an-1<1. Moreover, from the AM-GM inequality we get
so an>an-1; the sequence increases from the index n0.
By the monotonicity and boundedness the sequence converges. We show that its limit is . Let
; from (2) we see that
(2A-1)2 0,
0,
therefore . Hence,
.
Define . This sequence decreases from the index n0, and it converges to 0. Transforming (1), for n>n0 we have
| (3) |
Summing up (3), for n>n0 we get a lower bound for xn:
| (4) |
In the rest of the solution we consider the properties of the sequence (n+1)xn. We distinguish two cases.
Case 1: the sequence (n+1)xn has a finite accumulation point. Since except for finitely many indices, all accumulation points lie in the interval
. It is well-known that there is a minimal accumulation point; this point is called as the limes inferior of the sequence. Denote by c the smallest accumulation point. Then there is a sequence
of indices such that
.
Since there is no smallest accumulation point than c, for all 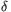 >0, by the Bolzano-Weierstrass theorem, (n+1)xn>c-
>0, by the Bolzano-Weierstrass theorem, (n+1)xn>c-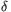 holds except for finitely many indices. Substuting this into (3) and summing up again, we obtain that for nk>n0 and
holds except for finitely many indices. Substuting this into (3) and summing up again, we obtain that for nk>n0 and 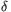
 c,
c,
From the transition k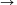
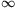 we get
we get
Then from 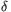
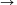 +0, we obtain
+0, we obtain
contradiction.
Case 2: The sequence (n+1)xn has no accumulation point. By the Bolzano-Weierstrass theorem and the positivity this means that for all real K, (n+1)xn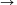
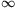 with finitely many exceptions, so (n+1)xn
with finitely many exceptions, so (n+1)xn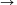
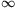 . Then there is an index n>n0 such that 1<nxn-1<(n+1)xn. Applyinig (3),
. Then there is an index n>n0 such that 1<nxn-1<(n+1)xn. Applyinig (3),
we get a contradiction, since both nxn-1-(n+1)xn and 1-2(n+1)xn are negative.
Remark. The term 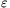 in the problem statement is important. Ommiting this quantity the statement would be false. For example, for the sequence
in the problem statement is important. Ommiting this quantity the statement would be false. For example, for the sequence ,
Statistics:
6 students sent a solution. 5 points: Ágoston Tamás, Mester Márton, Omer Cerrahoglu, Strenner Péter. 1 point: 2 students.
Problems in Mathematics of KöMaL, January 2012
