Problem A. 553. (January 2012)

A. 553. Suppose that in a simple graph G with n vertices the minimal degree 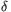(G) is at least 3n/4. Prove that for any 2-coloring of the edges of G, there is a connected subgraph with at least 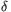(G)+1 vertices whose edges have the same color.

(Schweitzer-competition, 2011)

(5 pont)

Deadline expired on February 10, 2012.

Statistics:

6 students sent a solution.
5 points:	Ágoston Tamás, Gyarmati Máté, Janzer Olivér, Mester Márton, Omer Cerrahoglu, Strenner Péter.

Problems in Mathematics of KöMaL, January 2012