Mathematical and Physical Journal
for High Schools
Issued by the MATFUND Foundation
Already signed up?
New to KöMaL?

Problem A. 556. (February 2012)

A. 556. Prove that for arbitrary real numbers a_1,\ldots,a_n there exist a real t such that

\sum_{i=1}^n \big|\sin(t-a_i)\big| \le \ctg\frac\pi{2n}.

(5 pont)

Deadline expired on March 12, 2012.

Solution. Define the function f(x) = \sum_{i=1}^n \big|\sin(x-a_i)\big| and let M = \min\big( f(a_1), \ldots, f(a_n) \big). We will show that M \le \ctg\frac\pi{2n}. Then it follows that at least one of the choices t=a1, ..., t=an proves the statement.

 

The role of a1,...,an is symmetric and the function |sin x| is periodic by 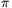\pi, so without loss of generality we may assume 0<a_1\le a_2\le\ldots\le a_n=\pi. Define a0=0 too; then f(a0)=f(an).

If a_1=\ldots=a_n=\pi, then f(a_1)=\ldots=f(a_n)=0, and the statement is trivial. In the rest of the solution we assume a1<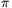\pi as well; then 0\le a_1-a_0,a_2-a_1,\ldots,a_n-a_{n-1}<\pi.

By the periodicity of |sin x|,

\int_0^\pi f = \sum_{i=1}^n \int_0^\pi \big|\sin(x-a_i)\big| \,\mathrm{d}x = \sum_{i=1}^n \int_0^\pi \big|\sin x\big| \,\mathrm{d}x = n \cdot 2 = 2n. (1)

Now we prove that

\int_{a_{k-1}}^{a_k} f = \big(f(a_{k-1})+f(a_k)\big) \cdot \tg\frac{a_k-a_{k-1}}2 (2)

for all 1\lek\len.

We prove (2) termwise. For each index 1\lei\len,

\int_{a_{k-1}}^{a_k} \sin(x-a_i) \,\mathrm{d}x = \cos(a_{k-1}-a_i) - \cos(a_k-a_i) = 2 \sin\frac{(a_{k-1}-a_i)+(a_k-a_i)}2 \sin\frac{a_k-a_{k-1}}2 =

= \bigg(2 \sin\Big(\frac{a_{k-1}+a_k}2-a_i\Big) \cos\frac{a_k-a_{k-1}}2\bigg) \tg\frac{a_k-a_{k-1}}2 = \Big( \sin(a_{k-1}-a_i) + \sin(a_k-a_i) \Big) \tg\frac{a_k-a_{k-1}}2.

In the interval [ak-1,ak] the function sin (x-ai) has constant sign: it is nonnegative for i\lek-1, and nonpositive for i\gek. Multiplying by (-1) for i<k and summing up we obtain (2).

Combining (1) and (2), and applying Jensen's inequality to the tangent function (which is convex in [0,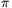\pi/2), we get

2n = \int_0^\pi f = \sum_{k=1}^n \int_{a_{k-1}}^{a_k} f = \sum_{k=1}^n \big(f(a_{k-1})+f(a_k)\big) \cdot \tg\frac{a_k-a_{k-1}}2 \ge

\ge 2M \cdot \sum_{k=1}^n \tg\frac{a_k-a_{k-1}}2 \ge 2M \cdot n \tg\bigg( \frac1n \sum_{k=1}^n \frac{a_k-a_{k-1}}2 \bigg) = 2nM \cdot \tg \frac\pi{2n},

M \le \ctg \frac\pi{2n}.

Statistics:

6 students sent a solution.
5 points:Gyarmati Máté, Janzer Olivér, Omer Cerrahoglu.
4 points:Machó Bónis.
0 point:2 students.

Problems in Mathematics of KöMaL, February 2012