 Problem A. 601. (November 2013)
Problem A. 601. (November 2013)
A. 601. Let q 1 be an integer. Prove that there is an integer Cq such that for every finite set A of integers |A+q.A|
1 be an integer. Prove that there is an integer Cq such that for every finite set A of integers |A+q.A| (q+1)|A|-Cq holds. (A+q.A is the set of those integers that can be expressed as a+qa' with some a,a'
(q+1)|A|-Cq holds. (A+q.A is the set of those integers that can be expressed as a+qa' with some a,a'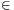 A.)
A.)
Schweitzer competition, 2013
(5 pont)
Deadline expired on December 10, 2013.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás. Első észrevételünk a következő.
1. lemma. Legyenek \(\displaystyle A\) és \(\displaystyle B\) egész számokból álló véges halmazok. Ekkor
\(\displaystyle |A+B|\ge |A|+|B|-1.\)
Bizonyítás. Jelölje \(\displaystyle A\) elemeit \(\displaystyle a_1<a_2<\dots<a_n\), valamint \(\displaystyle B\) elemeit \(\displaystyle b_1<b_2<\dots<b_m\). Ekkor
\(\displaystyle a_1+b_1<a_2+b_1<a_2+b_2<\dots<a_{n-1}+b_m<a_n+b_m\)
felsorol \(\displaystyle n+m-1\) különböző elemet \(\displaystyle A+B\)-ben. (Mindegy, hogy a tagok indexeit hogyan növeljük.) \(\displaystyle \blacksquare\)
Hogyha maradékosztályokra bontunk modulo \(\displaystyle q\), az alábbi erősítést kapjuk.
2. lemma. Amennyiben \(\displaystyle A\) tartalmaz \(\displaystyle r\)-féle maradékot modulo \(\displaystyle q\), teljesül, hogy
\(\displaystyle |A+q\cdot B|\ge r|B|+(|A|-r).\)
Bizonyítás. Jelölje \(\displaystyle a_1,a_2,\dots,a_r\) a legkisebb értéket az egyes maradékosztályokban. Ekkor \(\displaystyle A+q\cdot B\) tartalmazza \(\displaystyle a_1+q\cdot B,a_2+q\cdot B,\dots,a_r+q\cdot B\) elemeit. Ez összesen \(\displaystyle r|B|\) elem, hiszen különböző maradékosztályokba tartoznak.
Ezenfelül, ha az \(\displaystyle a_i\) mod \(\displaystyle q\) maradékosztályban szereplő elemek \(\displaystyle a_i=a_{i,1}<a_{i,2}<\dots<a_{i,k}\), és \(\displaystyle \max B=b_{max}\), akkor
\(\displaystyle \max(a_i+q\cdot B)=a_i+qb_{max}<a_{i,2}+qb_{max}<\dots<a_{i,k}+qb_{max}\)
további \(\displaystyle k-1\) elemét adja \(\displaystyle A+q\cdot B\)-nek, mely az \(\displaystyle a_i\) mod \(\displaystyle q\) maradékosztályba tartozik. Összesítve, ez \(\displaystyle (|A|-r)\)-rel javít a becslésen. \(\displaystyle \blacksquare\)
Térjünk rá a feladatra, rekurzív megközelítéssel.
1. észrevétel. Ha kiveszünk \(\displaystyle A\)-ból egy maradékosztályt, tehát az \(\displaystyle (i+q\mathbb{Z})\cap A=A_i\) halmaz elemeit, akkor a maradékokat megkülönböztetve
\(\displaystyle |A+q\cdot A|\ge |A_i+q\cdot A|+|(A\setminus A_i)+q\cdot (A\setminus A_i)|.\)
Itt az 1. lemma szerint fennáll, hogy \(\displaystyle |A_i+q\cdot A|\ge |A_i|+|q\cdot A|-1=|A_i|+|A|-1\).
2. észrevétel. Maradékosztályokra bontva,
\(\displaystyle |A+q\cdot A|\ge \sum_{i=0}^{q-1} |A_i+q\cdot A|\ge \sum_{i=0}^{q-1} |A_i+q\cdot A_i|.\)
Legyen \(\displaystyle A_i=i+q\cdot B_i\), ekkor \(\displaystyle |A_i+q\cdot A_i|=|B_i+q\cdot B_i|\). Amennyiben \(\displaystyle B_i\)-ben minden mod \(\displaystyle q\) maradék előfordul, \(\displaystyle |B_i+q\cdot B_i|\ge (q+1)|B_i|-q\) (2. lemma). Tehát amennyiben minden \(\displaystyle B_i\) vagy üres, vagy tartalmaz minden mod \(\displaystyle q\) maradékot:
\(\displaystyle |A+q\cdot A|\ge \sum_{B_i\neq \emptyset} (q+1)|B_i|-q\ge (q+1)|A|-q^2.\)
3. észrevétel. Erősíthető az 1. észrevétel, ha szemügyre vesszük \(\displaystyle A_i+q\cdot A\) azon elemeit, melyeket \(\displaystyle A_i+q\cdot A_i\) nem tartalmaz. Tekintsük az \(\displaystyle a+q\cdot A^*\) halmazt, ahol \(\displaystyle a\in A_i\) és \(\displaystyle A^*\subset A\setminus A_i\). Ha ez valamely \(\displaystyle a\in A_i\)-re diszjunkt \(\displaystyle A_i+q\cdot A_i\)-től, akkor
\(\displaystyle |A_i+q\cdot A|\ge |A_i+q\cdot A_i|+|A^*|.\)
Egyéb esetben minden \(\displaystyle a\in A_i\)-hez létezik \(\displaystyle a^*\in A^*\) és \(\displaystyle a',a''\in A_i\), melyre \(\displaystyle a+qa^*=a'+qa''\). Vagyis minden \(\displaystyle b\in B_i\)-hez létezik \(\displaystyle b'\in B_i\), melyre \(\displaystyle b+a^*=b'+a''\).
Alkalmazzuk ezt \(\displaystyle A^*=A_j=(j+q\mathbb{Z})\cap A\) választással valamely \(\displaystyle j\neq i\)-re. Tehát
\(\displaystyle |A_i+q\cdot A|\ge |A_i+q\cdot A_i|+\min_{j\neq i}|A_j|,\)
egyébiránt minden \(\displaystyle b\in B_i\)-hez létezik \(\displaystyle b'\in B_i\), melyre \(\displaystyle b'\equiv b+(j-i)\pmod q\). Hogyha a lehetséges \(\displaystyle (j-i)\) értékek legnagyobb közös osztója \(\displaystyle 1\), kapjuk, hogy \(\displaystyle B_i\)-ben minden mod \(\displaystyle q\) maradék előfordul, és a 2. észrevétel alkalmazható. Ha pedig \(\displaystyle d>1\) közös osztó, akkor nézhetjük \(\displaystyle A\) helyett a kisebb átmérőjű \(\displaystyle (A-i)/d\subset \mathbb{Z}\) halmazt.
Eredmény. Legyen \(\displaystyle f(n)=\min\{|A+q\cdot A|:|A|=n\}\), és jelölje \(\displaystyle m\) a legkisebb nem üres \(\displaystyle A_i\) nagyságát. Ekkor vagy \(\displaystyle f(n)\ge (q+1)n-q^2\), vagy pedig
\(\displaystyle f(n)\ge \max \{ m+n-1+f(n-m),m+f(m)+f(n-m)\}.\)
Következmény. \(\displaystyle f(n)\ge \max \left\{\frac{\mu}{q+1}n-q^22^{\mu-3(q+1)}|\mu=3(q+1),3(q+1)+1,\dots,(q+1)^2\right\}\) (lásd a hivatalos megoldást).
Statistics:
2 students sent a solution. 0 point: 2 students.
Problems in Mathematics of KöMaL, November 2013
