Problem B. 4344. (March 2011)

B. 4344. The lengths of the parallel sides of a symmetric trapezium are a and c. The midpoints of the legs are E and F. Let G denote the orthogonal projection of point E on the line of the leg BC. What is the area of the trapezium if point C divides the line segment GF in a 1:2 ratio? (C lies closer to point G.)

(4 pont)

Deadline expired on April 11, 2011.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás. A feladat szövege alapján világos, hogy az \(\displaystyle E\) és \(\displaystyle F\) pontok rendre az \(\displaystyle AD\), illetve a \(\displaystyle BC\) szár felezőpontjai, továbbá az \(\displaystyle AB\) alap hosszabb mint a \(\displaystyle CD\) alap. Ezek szerint \(\displaystyle a\ne c\). Tegyük fel, hogy \(\displaystyle a>c\). A szárak hossza legyen \(\displaystyle 4x\), ekkor \(\displaystyle FG=3x\). Ha a \(\displaystyle C\) csúcsnak az \(\displaystyle AB\) alapra eső merőleges vetülete \(\displaystyle H\), akkor \(\displaystyle EF=\frac{a+c}{2}\) és \(\displaystyle BH=\frac{a-c}{2}\). Az \(\displaystyle EFG\) és \(\displaystyle CBH\) derékszögű háromszögek hasonló volta miatt

\(\displaystyle 12x^2=CB\cdot FG=EF\cdot BH=\frac{a+c}{2}\cdot \frac{a-c}{2} =\frac{a^2-c^2}{4}.\)

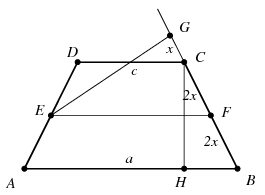
Ezek alapján a trapéz területe

\(\displaystyle EF\cdot CH=EF\cdot\sqrt{CB^2-BH^2}= \frac{a+c}{2}\cdot \sqrt{(4x)^2-\left(\frac{a-c}{2}\right)^2}\)

\(\displaystyle =\frac{a+c}{2}\cdot \sqrt{\frac{a^2-c^2}{3}-\left(\frac{a-c}{2}\right)^2} =\frac{a+c}{2}\cdot \sqrt{\frac{a^2+6ac-7c^2}{12}}\)

\(\displaystyle =\sqrt{\frac{(a+c)^2(a+7c)(a-c)}{48}}.\)

Statistics:

87 students sent a solution.
4 points:	62 students.
3 points:	8 students.
2 points:	3 students.
1 point:	7 students.
0 point:	5 students.
Unfair, not evaluated:	2 solutionss.

Problems in Mathematics of KöMaL, March 2011