 Problem B. 4586. (December 2013)
Problem B. 4586. (December 2013)
B. 4586. K, L and M are points of sides AB, AC and BC of triangle ABC, respectively. KL is parallel to BC, KL=LC, and . Prove that LM=AK.
(Kvant)
(4 pont)
Deadline expired on January 10, 2014.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás. Mivel \(\displaystyle KL||BC\) és \(\displaystyle KL=LC\), láthatjuk, hogy \(\displaystyle KCB\angle = CKL\angle = LCK\angle\), és így \(\displaystyle CK\) az \(\displaystyle ACB\angle\) felezője.
Legyen \(\displaystyle N\) az a pont az \(\displaystyle AB\) egyenesen, amire \(\displaystyle KN\) párhuzamos \(\displaystyle LM\)-mel. Ekkor tehát \(\displaystyle KL||MN\) és \(\displaystyle KN||LM\), vagyis a \(\displaystyle KLMN\) négszög paralelogramma. Mivel \(\displaystyle CNK\angle=CML\angle=180^\circ-LMB\angle=180^\circ-KAC\angle\), az \(\displaystyle AKNC\) négyszög húrnégyszög.
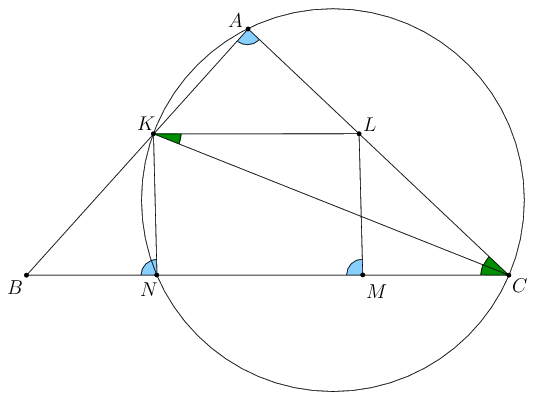
Mivel \(\displaystyle CK\) az \(\displaystyle ACN\) szög felezője, az \(\displaystyle AKNC\) négyszög köré írt körön az \(\displaystyle AK\) és \(\displaystyle KN\) ívek ugyanakkora kerületi szöghöz tartoznak, így \(\displaystyle AK=KN\). Mivel pedig \(\displaystyle KLMN\) paralelogramma, \(\displaystyle KN=LM\). Tehát,
\(\displaystyle AK = KN = LM. \)
Statistics:
144 students sent a solution. 4 points: 125 students. 3 points: 14 students. 0 point: 5 students.
Problems in Mathematics of KöMaL, December 2013
