Problem B. 4910. (November 2017)

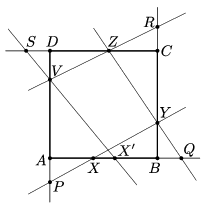
B. 4910. Let \(\displaystyle P\), \(\displaystyle Q\), \(\displaystyle R\) and \(\displaystyle S\) denote points on the lines of the sides of a square \(\displaystyle ABCD\) such that \(\displaystyle AP=BQ=CR=DS\), as shown in the figure. Starting from an arbitrary interior point \(\displaystyle X\) of side \(\displaystyle AB\), let the line \(\displaystyle PX\) intersect line \(\displaystyle BC\) at \(\displaystyle Y\), let \(\displaystyle QY\) intersect line \(\displaystyle CD\) at \(\displaystyle Z\), let \(\displaystyle RZ\) intersect line \(\displaystyle DA\) at \(\displaystyle V\), and finally let \(\displaystyle SV\) intersect line \(\displaystyle AB\) at \(\displaystyle X'\). Prove that if \(\displaystyle X'\) and \(\displaystyle X\) coincide then \(\displaystyle XYZV\) is a square.

(5 pont)

Deadline expired on December 11, 2017.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás. Legyen a négyzet oldala egységnyi és \(\displaystyle AP=BQ=CR=DS=s\), \(\displaystyle AX=t\) és \(\displaystyle BY=f(t)\).

Ekkor \(\displaystyle PAX\) és \(\displaystyle YBX\) egymáshoz hasonló derékszögű háromszögek, hiszen \(\displaystyle AXP\sphericalangle = BXY\sphericalangle\) hegyesszögeik csúcsszögek. Így \(\displaystyle AX:AP=XB:YB\), azaz \(\displaystyle \dfrac{t}{s}=\dfrac{1-t}{f(t)}\) szerint \(\displaystyle f(t)=s\dfrac{1-t}{t}=\dfrac{s}{t}-s\). Ugyanígy

\(\displaystyle CZ=f(BY)=f(f(t))=\dfrac{s}{\dfrac{s}{t}-s}-s=\dfrac{1}{\dfrac{1}{t}-1}-s= \dfrac{t}{1-t}-s=-(s+1)+\dfrac{1}{1-t}, \)

\(\displaystyle AX'=f(DV)=f(f(CZ))=-(s+1)+\dfrac{1}{1-CZ}= -(s+1)+\dfrac{1}{1-\left(-(s+1)+\dfrac{1}{1-t}\right)}= \)

\(\displaystyle =-(s+1)+\dfrac{1-t}{1-t-t+s(1-t)}=-(s+1)+\dfrac{1-t}{(s+1)-(s+2)t}. \)

Ha \(\displaystyle X'\) és \(\displaystyle X\) egybeesnek, akkor \(\displaystyle AX'=AX\), azaz \(\displaystyle -(s+1)+\dfrac{1-t}{(s+1)-(s+2)t}=t\). Ez \(\displaystyle t\)-ben másodfokú egyenletre vezet:

\(\displaystyle -(s+1)((s+1)-(s+2)t)+(1-t)=t((s+1)-(s+2)t), \)

\(\displaystyle (s+2)t^2 + (s^2+2s)t -(s^2+2s) =0, \)

\(\displaystyle (s+2)(t^2 + st -s)=0. \)

Mivel \(\displaystyle s>0\), azért az egyenlet egyetlen pozitív megoldása \(\displaystyle t_1=\dfrac{-s+\sqrt{s^2+4s}}{2}\). Ezzel

\(\displaystyle BY=f(t_1)=\dfrac{s}{t_1}-s=\dfrac{2s}{-s+\sqrt{s^2+4s}}-s= \dfrac{2s(s+\sqrt{s^2+4s})}{4s}-s=t_1, \)

vagyis \(\displaystyle BY=f(AX)=AX\), ugyanígy \(\displaystyle CZ=f(BY)=BY\), \(\displaystyle DV=f(CZ)=CZ\) és \(\displaystyle AX=AX'=f(DV)=DV\). Ebből következik, hogy az \(\displaystyle ABCD\) négyzet középpontja körüli 90 fokos elforgatás az egész alakzatot, így az \(\displaystyle XYZV\) négyszöget is önmagára képezi, tehát \(\displaystyle XYZV\) valóban négyzet.

Statistics:

69 students sent a solution.
5 points:	Beke Csongor, Busa 423 Máté, Csépányi István, Csizmadia Viktória, Daróczi Sándor, Deák Bence, Dobák Dániel, Döbröntei Dávid Bence, Fekete Richárd, Fülöp Anna Tácia, Füredi Erik Benjámin, Gáspár Attila, Gyetvai Miklós, Hervay Bence, Jánosik Áron, Janzer Orsolya Lili, Jedlovszky Pál, Kerekes Anna, Kószó Máté József, Lukács Lilla Réka, Márton Dénes, Molnár-Sáska Zoltán, Nagy Nándor, Noszály Áron, Pituk Gábor, Póta Balázs, Saár Patrik, Schifferer András, Schrettner Jakab, Shuborno Das, Soós 314 Máté, Szabó 417 Dávid, Szabó 991 Kornél, Szabó 997 Balázs István, Tiderenczl Dániel, Tiszay Ádám, Tubak Dániel, Vári-Kakas Andor, Várkonyi Zsombor, Velkey Vince, Weisz Máté, Zólomy Kristóf, Zsigri Bálint.
4 points:	8 students.
2 points:	2 students.
1 point:	3 students.
0 point:	13 students.

Problems in Mathematics of KöMaL, November 2017