Problem B. 5297. (February 2023)

B. 5297. In a triangle \(\displaystyle ABC\), \(\displaystyle \angle BAC =2\angle CBA\). Let \(\displaystyle A'\), \(\displaystyle B'\), \(\displaystyle C'\) denote interior points of sides \(\displaystyle CA\), \(\displaystyle AB\) and \(\displaystyle BC\), respectively, such that triangle \(\displaystyle A'B'C'\) is similar to triangle \(\displaystyle ABC\). Show that the angle bisectors of angles \(\displaystyle BAC\) and \(\displaystyle B'A'C'\) intersect each other on the line segment \(\displaystyle B'C'\).

Proposed by G. Kós, Budapest

(4 pont)

Deadline expired on March 10, 2023.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás. Legyen \(\displaystyle M\) a \(\displaystyle B'C'\) szakasz és a \(\displaystyle B'A'C'\) szög felezőjének metszéspontja; azt kell igazolnunk, hogy \(\displaystyle AM\) felezi a \(\displaystyle BAC\) szöget.

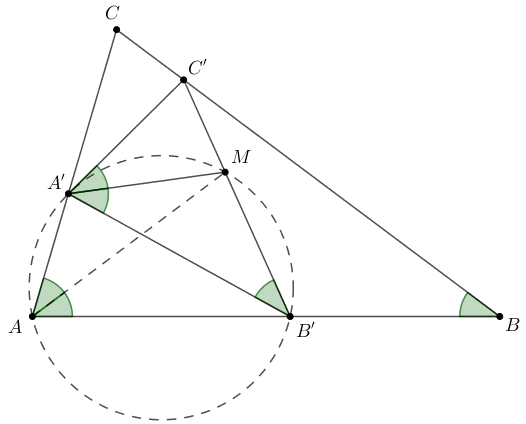
A feltétel szerint \(\displaystyle ABC\triangle\sim A'B'C'\triangle\) és \(\displaystyle BAC\sphericalangle=2CBA\sphericalangle\), ezért

\(\displaystyle MB'A'\sphericalangle =C'B'A'\sphericalangle =CBA\sphericalangle =\frac12BAC\sphericalangle =\frac12B'A'C'\sphericalangle =B'A'M\sphericalangle =MA'C'\sphericalangle. \)

Az \(\displaystyle A'B'M\) háromszög szögeit összeszámolva láthatjuk, hogy

\(\displaystyle 180^\circ-A'MB'\sphericalangle =B'A'M\sphericalangle+MB'A'\sphericalangle =\frac12BAC\sphericalangle+\frac12BAC\sphericalangle =BAC\sphericalangle =B'AA'\sphericalangle, \)

ezért \(\displaystyle AB'MA'\) húrnégyszög.

Végül, az \(\displaystyle AB'MA'\) húrnégyszögben

\(\displaystyle BAM\sphericalangle = B'AM\sphericalangle = B'A'M\sphericalangle = MB'A'\sphericalangle = MAA'\sphericalangle = MAC\sphericalangle, \)

vagyis \(\displaystyle AM\) valóban felezi a \(\displaystyle BAC\) szöget.

Statistics:

70 students sent a solution.
4 points:	Ali Richárd, Aravin Peter, Balaskó Imola, Bodor Mátyás, Bui Thuy-Trang Nikolett, Chrobák Gergő, Csupor Albert Dezső, Diaconescu Tashi, Domján Olivér, Fodor Dóra, Hosszu Noel, Inokai Ádám, Kerekes András, Kocsis 827 Péter, Kosztolányi Karina, Kovács Benedek Noel, Melján Dávid Gergő, Nagy 292 Korina, Nagy 429 Leila, Nguyen Kim Dorka, Pálfi András, Pletikoszity Martin, Romaniuc Albert-Iulian, Sárdinecz Dóra, Sütő Áron, Szabó Zóra, Szittyai Anna, Tarján Bernát, Teveli Jakab, Tusnády Sámuel, Varga Boldizsár, Veres Dorottya, Vigh 279 Zalán, Virág Lénárd Dániel, Virág Rudolf, Zhai Yu Fan.
3 points:	21 students.
2 points:	6 students.
1 point:	2 students.
0 point:	3 students.

Problems in Mathematics of KöMaL, February 2023