 Problem C. 1146. (December 2012)
Problem C. 1146. (December 2012)
C. 1146. Given one vertex and the midpoints of all the sides of a pentagon on the plane, prove that it is always possible to find a point on the plane, such that it forms a parallelogram with three of the six given points, and another parallelogram with the other three points.
(5 pont)
Deadline expired on January 10, 2013.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás. Legyen az ötszög csúcsa \(\displaystyle A\), az ebből a csúcsból kiinduló oldalak felezőpontjai \(\displaystyle P\) és \(\displaystyle Q\), a további oldalak felezőpontjai pedig \(\displaystyle K\), \(\displaystyle L\), és \(\displaystyle M\). Az ötszög \(\displaystyle A\) csúcsával szomszédos két csúcsot jelölje \(\displaystyle B\) és \(\displaystyle E\). Megmutatjuk, hogy a \(\displaystyle BE\) szakasz \(\displaystyle R\) felezőpontja megfelelő pont.
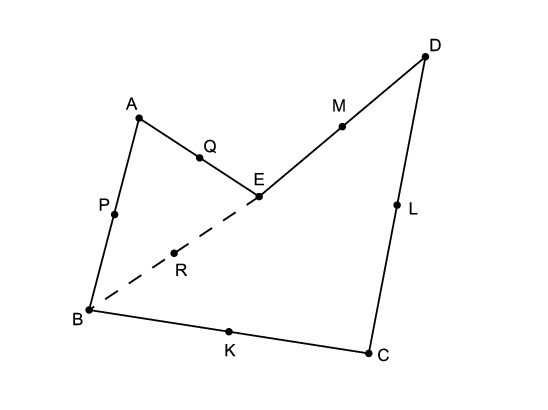
Az \(\displaystyle ABE\) háromszögben a \(\displaystyle P\), \(\displaystyle Q\), és \(\displaystyle R\) pontok oldalfelező pontok, így a középvonalak miatt az \(\displaystyle APQR\) négyszög paralelogramma. Az \(\displaystyle RKLM\) négyszög szintén paralelogramma, mert egy négyszög oldalainak felezőpontjai paralelogrammát határoznak meg.
Statistics:
166 students sent a solution. 5 points: 123 students. 4 points: 14 students. 3 points: 6 students. 2 points: 4 students. 1 point: 7 students. 0 point: 9 students. Unfair, not evaluated: 3 solutionss.
Problems in Mathematics of KöMaL, December 2012
