 Problem C. 1199. (December 2013)
Problem C. 1199. (December 2013)
C. 1199. The accompanying figure shows a sequence of designs made up of floor tiles. The number of dark grey tiles in the designs is 1,6,13,24,37,..., respectively.
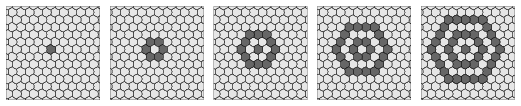
Sophie proved that the number of dark grey tiles in the designs with odd indices in the series is a quadratic function of the index. Determine what number of dark grey tiles there are in the ninety-ninth design according to Sophie's formula.
(5 pont)
Deadline expired on January 10, 2014.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás. Legyen \(\displaystyle f(n)=an^2+bn+c\) a keresett másodfokú függvény hozzárendelési szabálya, ahol \(\displaystyle n\) jelöli a páratlan sorszámot. Tudjuk, hogy \(\displaystyle f(1)=1\), \(\displaystyle f(3)=13\), \(\displaystyle f(5)=37\), ezért a következő egyenletrendszert kell megoldanunk: \(\displaystyle a+b+c=1\), \(\displaystyle 9a+3b+c=13\), \(\displaystyle 25a+5b+c=37\). A megoldás: \(\displaystyle a=1,5\), \(\displaystyle b=0\), \(\displaystyle c=-0,5\). A másodfokú függvény: \(\displaystyle f(n)=1,5n^2-0,5\). Vagyis Zsófi a kilencvenkilencedik mintán lévő szürke lapok számára ezt kapta: \(\displaystyle f(99)=1,5\cdot99^2-0,5=14 701\).
Statistics:
182 students sent a solution. 5 points: 120 students. 4 points: 15 students. 3 points: 22 students. 2 points: 10 students. 1 point: 11 students. 0 point: 3 students. Unfair, not evaluated: 1 solutions.
Problems in Mathematics of KöMaL, December 2013
