 Problem C. 827. (November 2005)
Problem C. 827. (November 2005)
C. 827. You are sitting in an aeroplane. Looking through a window 20 cm from your eye, you can see two boats in the direction of each of the lower corners of the 25 cm×40 cm window, respectively. Given that the plane is flying at an altitude of 10.3 km, and your eye is level with the horizontal bimedian of the window, determine the distance between the two boats.
(5 pont)
Deadline expired on December 15, 2005.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás: Készítsünk ábrát!
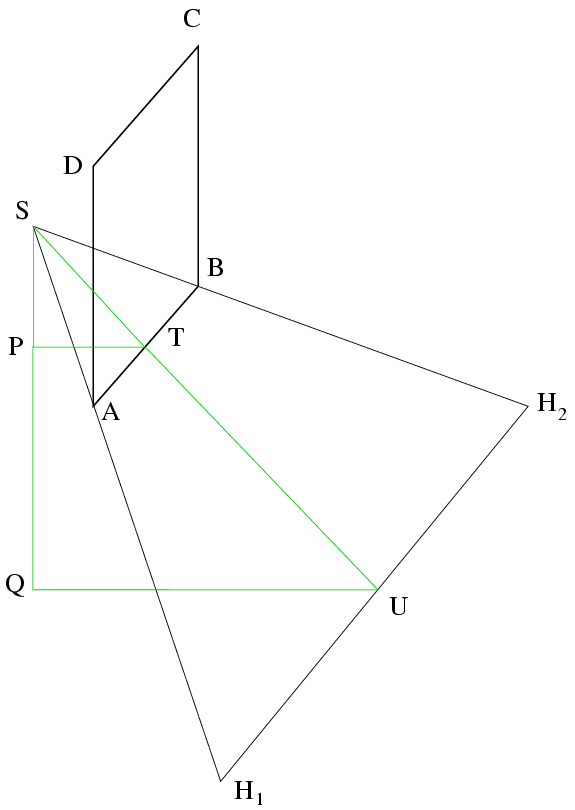
;
;
;
;
cm =20 cm; PT=20 cm; AB=40 cm; SQ=10,3 km.
, így
, vagyis
. Mivel
, ezért
, ezt az előbbi kifejezésbe beírva, majd a megfelelő értékeket behelyettesítve:
Látható, hogy a két hajó távolsága független a szemünk ablaktól való távolságától és vízszintes helyzetétől.
Statistics:
340 students sent a solution. 5 points: 284 students. 4 points: 3 students. 3 points: 7 students. 2 points: 14 students. 1 point: 14 students. 0 point: 16 students. Unfair, not evaluated: 2 solutionss.
Problems in Mathematics of KöMaL, November 2005
