 Problem C. 874. (November 2006)
Problem C. 874. (November 2006)
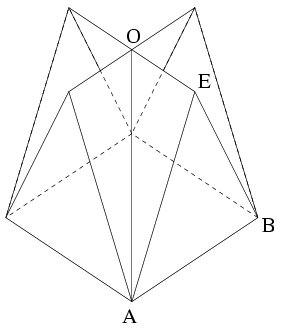
C. 874. The roof of a newsstand with a square base 3 m on a side consists of two regular triangular prisms that intersect each other. One lateral face of each prism coincides with the ceiling of the newsstand. (The prisms are rotated through 90o relative to each other.) Calculate the surface area of the roof.
(5 pont)
Deadline expired on December 15, 2006.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás. A tetőfelület nyolc egybevágó, derékszögű háromszögből tevődik össze. Számítsuk ki egy ilyennek a területét:
A tetőfelület nagysága tehát 8.0,5.4,5 m2=18 m2.
Ha a felületbe beleszámítjuk a négy egybevágó, 3 m oldalú szabályos háromszög területét is, akkor nagysága .
Statistics:
353 students sent a solution. 5 points: 230 students. 4 points: 76 students. 3 points: 12 students. 2 points: 11 students. 1 point: 6 students. 0 point: 17 students. Unfair, not evaluated: 1 solutions.
Problems in Mathematics of KöMaL, November 2006
