 Problem C. 907. (September 2007)
Problem C. 907. (September 2007)
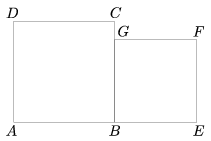
C. 907. The square ABCD of side a and the square BEFG of side b are drawn next to each other as shown in the figure. Express in terms of a and b the area of the quadrilateral formed by the midpoints of the line segments AB, BE, FC and DG.
(5 pont)
Deadline expired on October 15, 2007.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás: F1F4 az ABGD trapéz középvonala, így párhuzamos AD-vel, és hossza .
F2F3 az EFCB trapéz középvonala, így párhuzamos BC-vel, hossza pedig .
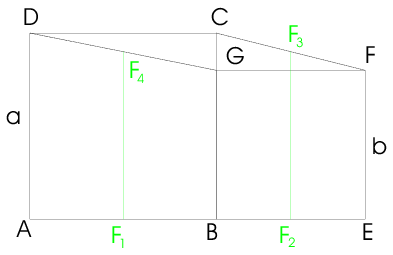
Mivel AD||BC, ezért F1F2F3F4 két szemben fekvő oldala, F1F4 és F2F3 párhuzamos és egyenlő, tehát ez a négyszög paralelogramma. Mivel oldalai merőlegesek egymásra (), ezért téglalap.
F1F2=F1B+BF2=a/2+b/2, tehát F1F2F3F4 négyzet. Így területe:
Statistics:
617 students sent a solution. 5 points: 168 students. 4 points: 84 students. 3 points: 71 students. 2 points: 84 students. 1 point: 69 students. 0 point: 131 students. Unfair, not evaluated: 10 solutionss.
Problems in Mathematics of KöMaL, September 2007
