Problem K. 272. (December 2010)

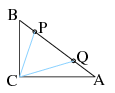
K. 272. P is a point on the hypotenuse AB of a right-angled triangle ABC, such that AC=AP. Q is a point on the line segment AP, such that . Prove that triangle CQB is isosceles.

(6 pont)

Deadline expired on January 10, 2011.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás. Jelöljük az \(\displaystyle A\) csúcsnál levő szöget \(\displaystyle \alpha\)-val és számoljuk ki a szögeket. Az \(\displaystyle APC\triangle\) egyenlőszárú a feladat szerint, csúcssöge \(\displaystyle \alpha\), ezért \(\displaystyle APC\angle = 90^\circ - \frac\alpha 2\). \(\displaystyle PCQ\triangle\)-ben \(\displaystyle PQC\angle=180^\circ-(45^\circ + 90^\circ - \frac\alpha 2)=45^\circ + \frac\alpha 2\). Ez a szög az egyik külső szöge \(\displaystyle QCA\triangle\)-nek, ezért \(\displaystyle QCA\angle=45^\circ + \frac\alpha 2 - \alpha=45^\circ - \frac\alpha 2\). \(\displaystyle QCB\angle\) az előző szög pótszöge, azaz \(\displaystyle QCB\angle=45^\circ + \frac\alpha 2\). Mivel a \(\displaystyle BCQ\triangle\)-ben a \(\displaystyle C\)-nél és a \(\displaystyle Q\)-nál levő szög megegyezik, ezért a \(\displaystyle BCQ\triangle\) egyenlőszárú.

Statistics:

185 students sent a solution.
6 points:	119 students.
5 points:	18 students.
4 points:	8 students.
3 points:	5 students.
2 points:	6 students.
1 point:	5 students.
0 point:	20 students.
Unfair, not evaluated:	4 solutionss.

Problems in Mathematics of KöMaL, December 2010