 Problem P. 4539. (April 2013)
Problem P. 4539. (April 2013)
P. 4539. The base of a solid 4r-high right cylinder is a circle of radius r. One side of the surface of a tale made of hard but flexible material is slippery, whilst on the other side the frictional coefficient is 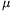 . The two parts are separated by a straight line. The cylinder is placed to the slippery side of the table (with its circular base on the tabletop), and is given a push perpendicularly to the separation line. Will the cylinder fall when it reaches the separation line or not, and if yes how, if a)
. The two parts are separated by a straight line. The cylinder is placed to the slippery side of the table (with its circular base on the tabletop), and is given a push perpendicularly to the separation line. Will the cylinder fall when it reaches the separation line or not, and if yes how, if a) 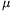 =0.2; b)
=0.2; b) 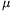 =0.7; c)
=0.7; c) 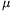 =1.4? (The rotational inertia of a cylinder of mass m, radius of r and height of 4r about a horizontal axis through its centre of mass is
=1.4? (The rotational inertia of a cylinder of mass m, radius of r and height of 4r about a horizontal axis through its centre of mass is .)
(6 pont)
Deadline expired on May 10, 2013.
Sorry, the solution is available only in Hungarian. Google translation
Megoldásvázlat. \(\displaystyle a)\) Ha \(\displaystyle \mu<\mu_1=\frac12\), akkor a henger nem billen fel, az alaplapján csúszva fokozatosan lefékeződik.
\(\displaystyle b)\) Ha \(\displaystyle \mu_1<\mu<\mu_2=\frac{31}{24}\left(\approx1{,}3\right)\), akkor a henger tömegközéppontjának mozgása fokozatosan fékeződik, s közben megemelkedik (előre billen), és ha elegendően nagy volt a kezdősebessége, felborul.
\(\displaystyle c)\) Ha \(\displaystyle \mu_2<\mu\), akkor a henger az érdes térfélen hirtelen lefékeződik, ,,megszorul'' (mintha egy kicsiny ütközőhöz érkezett volna), és az asztallal érintkező pontja körül elfordulva előre billen. (Ha elegendően nagy volt a kezdősebessége, a henger felborul.)
Statistics:
9 students sent a solution. 5 points: Fehér Zsombor, Sárvári Péter. 4 points: 1 student. 3 points: 2 students. 2 points: 2 students. 1 point: 2 students.
Problems in Physics of KöMaL, April 2013
