Problem P. 5069. (November 2018)

P. 5069. A solid cylinder of mass \(\displaystyle M\) and of radius \(\displaystyle R\) was placed onto a slope of angle of inclination of \(\displaystyle \alpha\). The cylinder is attached to the top end of the inclined plane by means of a horizontal thread as shown in the figure. Next to the object there is another cylinder of mass \(\displaystyle m\) and of radius \(\displaystyle r\). Friction between the two cylinders is negligible, and the cylinder of mass \(\displaystyle M\) is not rising. What is the least value of the coefficient of static friction between the slope and the cylinder of radius \(\displaystyle R\) if the cylinders do not slide on the slope?

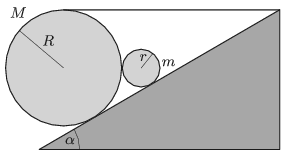
Data: \(\displaystyle \alpha=30^\circ\), \(\displaystyle R=3r\), \(\displaystyle M=3m\).

(5 pont)

Deadline expired on December 10, 2018.

Sorry, the solution is available only in Hungarian. Google translation

Megoldás. A megadott sugárarányok esetén a hengerek tengelyére illeszkedő sík

\(\displaystyle \varphi=\arcsin\frac{R-r}{R+r}=\arcsin\frac{1}{2}=30^\circ\)

nagyságú szöget zár be a lejtő síkjával, tehát a hengerek tengelye azonos magasságban helyezkedik el. A hengerekre az ábrán látható erők hatnak:

Itt már kihasználtuk, hogy a két henger között nincs súrlódás, tehát a közöttük fellépő erő vízszintes. Az \(\displaystyle M\) tömegű hengerre ható eredő forgatónyomaték nulla, emiatt a fonálban ébredő erő megegyezik az \(\displaystyle S\) súrlódási erővel. Az \(\displaystyle m\) tömegű hengerre sem hat forgatónyomaték, ami csak úgy lehetséges, ha a lejtő és ezen henger között nem lép fel súrlódási erő.

Az erők egyensúlyának feltétele:

\(\displaystyle F\,\frac{\sqrt{3}}{2}=mg,\)

\(\displaystyle \frac{1}{2}F=K,\)

\(\displaystyle Mg=\frac{1}{2}S+\frac{\sqrt{3}}{2}N,\)

\(\displaystyle K+\frac{1}{2}N=\left(1+ \frac{\sqrt{3}}{2} \right)S.\)

Ennek az egyenletrendszernek a megoldása:

\(\displaystyle F=\frac{2}{\sqrt{3}}mg,\)

\(\displaystyle K=\frac{1}{\sqrt{3}}mg,\)

\(\displaystyle S=\frac{(M+m)g}{2+\sqrt{3}},\)

\(\displaystyle N=(M+m)g-\frac{2}{\sqrt{3}}mg.\)

Az \(\displaystyle M\) tömegű henger akkor nem emelkedik fel a lejtőről, ha \(\displaystyle N\ge 0\), vagyis ha

\(\displaystyle M\ge \left(\frac{2}{\sqrt{3}}-1\right)m\approx 0{,}15\,m.\)

A megadott \(\displaystyle M=3m\) esetén ez teljesül. Az \(\displaystyle M\) tömegű henger akkor nem csúszik meg a lejtőn, ha (\(\displaystyle M=3m\) tömegarány mellett) a súrlódási együttható

\(\displaystyle \mu \ge \frac{S}{N} \approx 0{,}38.\)

Statistics:

64 students sent a solution.
5 points:	Békési Ábel, Bokor Endre, Bukor Benedek, Csépányi István, Fekete Levente, Fiam Regina, Fülöp Sámuel Sihombing, Hartmann Alice, Havasi Márton, Horváth 127 Ádám, Jánosik Áron, Keltai Dóra, Laposa Hédi, Lipták Gergő, Mácsai Dániel, Marozsák Tádé, Máth Benedek, Merkl Gergely, Molnár Mátyás, Morvai Orsolya, Olosz Adél, Osztényi József, Pácsonyi Péter, Rusvai Miklós, Sal Dávid, Sas 202 Mór, Schneider Anna, Sugár Soma, Szoboszlai Szilveszter, Tafferner Zoltán, Telek Dániel, Tiefenbeck Flórián, Toronyi András, Turcsányi Máté, Varga Vázsony, Vaszary Tamás.
4 points:	15 students.
3 points:	9 students.
2 points:	1 student.
1 point:	2 students.
0 point:	1 student.

Problems in Physics of KöMaL, November 2018