 Problem P. 5546. (February 2024)
Problem P. 5546. (February 2024)
P. 5546. In the Eskimos' Palace of Wonders, there is an igloo of radius of \(\displaystyle R=3~\text{m}\) which is built on a circular ice ink, rotating at an angular speed of \(\displaystyle \omega=\pi/6~\text{s}^{-1}\) around a vertical axis. Two children are sitting in the rotating igloo in the position shown in the figure, at an angle of \(\displaystyle \varphi=120^\circ\) with respect to each other. The child sitting at \(\displaystyle A\) manages to launch the puck so that it arrives at the hand of the other child sitting at \(\displaystyle B\), in a time of \(\displaystyle t=2~\text{s}\) after the launch.
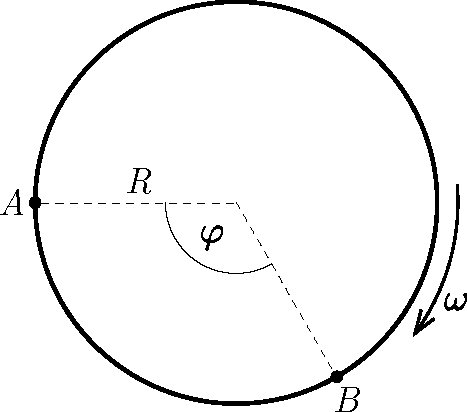
\(\displaystyle a)\) With respect to the igloo at what speed and in what direction did the child at \(\displaystyle A\) have to start the puck?
\(\displaystyle b)\) What distance does the puck approach the centre of the igloo as it moves?
(5 pont)
Deadline expired on March 18, 2024.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás. \(\displaystyle a)\) Az iglu \(\displaystyle t=2\,\mathrm{s}\) idő alatt \(\displaystyle \alpha=\omega t=60^\circ\)-ot fordul el, tehát (az inerciarendszerből nézve) a \(\displaystyle B\) pont a \(\displaystyle B'\) pontba kerül. A korong – súrlódás hiányában – az inerciarendszerben egyenes vonalú egyenletes mozgást végez, és \(\displaystyle t\) idő alatt a \(\displaystyle \overline{AB'}=R\) távolságot kell megtennie (\(\displaystyle \varphi-\alpha=60^\circ\)). Eszerint a sebesség nagysága az inerciarendszerből nézve
\(\displaystyle v_\mathrm{ir}=\frac{R}{t}=1{,}5\,\mathrm{m/s},\)
iránya pedig az \(\displaystyle A\) ponthoz húzott sugárral \(\displaystyle 60^\circ\)-os szöget zár be (1. ábra).
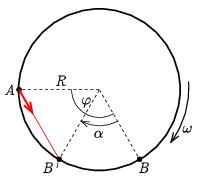
1. ábra
Az inerciarendszerből nézve az \(\displaystyle A\) pont \(\displaystyle v_A=R\omega=\pi/2\,\mathrm{m/s}\) sebességgel mozog, az ellökés pillanatában a rajzon függőlegesen felfelé. A korong sebességvektora az igluból nézve
\(\displaystyle \boldsymbol{v}=\boldsymbol{v}_\mathrm{ir}-\boldsymbol{v}_A.\)
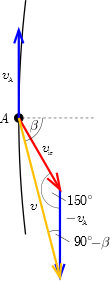
2. ábra
A sebességvektor nagysága a koszinusztétel alapján (2. ábra):
\(\displaystyle v=\sqrt{v_\mathrm{ir}^2+v_A^2-2v_\mathrm{ir}v_A\cos150^\circ}=2{,}97\,\mathrm{m/s},\)
az \(\displaystyle A\) ponthoz húzott sugárral bezárt \(\displaystyle \beta\) szöge pedig a szinusztétel alapján:
\(\displaystyle \frac{\sin(90^\circ-\beta)}{\sin150^\circ}=\frac{v_\mathrm{ir}}{v}\qquad\rightarrow\qquad\beta=75{,}4^\circ.\)
\(\displaystyle b)\) A korong az iglu középpontjához legközelebb a mozgása felénél lesz. A pont középponttól mért távolsága ugyanakkora az igluból és az inerciarendszerből nézve is. A távolság könnyen leolvasható az 1. ábráról, ami az \(\displaystyle R\) oldalú szabályos háromszög magassága:
\(\displaystyle d_\mathrm{min}=\frac{\sqrt{3}}{2}R=2{,}6\,\mathrm{m}.\)
Megjegyzés. Nem része a feladatnak, de számítógéppel kirajzolható a pályagörbe az igluból nézve (a korong iglu jegén hagyott nyoma). Ha felveszünk egy derékszögű koordináta-rendszert, melynek középpontja az iglu középpontja, és az \(\displaystyle A\) pont koordinátái (\(\displaystyle -R\) , 0), akkor az inerciarendszerben könnyen felírhatjuk a korong koordinátáit az idő függvényében:
$$\begin{align*} x_\mathrm{ir}&=-R+\frac{v_\mathrm{ir}}{2}t,\\ y_\mathrm{ir}&=\frac{-\sqrt{3}v_\mathrm{ir}}{2}t. \end{align*}$$Az iglu állandó \(\displaystyle -\omega\) szögsebességgel elfordul az inerciarendszerhez képest, így az igluból nézve az inerciarendszer forog \(\displaystyle \omega\) szögsebességgel. Az igluhoz rögzített koordináta-rendszerben (amely az ellökés pillanatában megegyezik az inerciarendszerbelivel) a \(\displaystyle t\) időpillanatban egy \(\displaystyle \omega t\) szögű forgatással kapjuk meg a korong koordinátáit:
$$\begin{align*} x&=x_\mathrm{ir}\cos(\omega t)-y_\mathrm{ir}\sin(\omega t)=\left(-R+\frac{v_\mathrm{ir}}{2}t\right)\cos(\omega t)+\frac{\sqrt{3}v_\mathrm{ir}}{2}t\sin(\omega t),\\ y&=x_\mathrm{ir}\sin(\omega t)+y_\mathrm{ir}\cos(\omega t)=\left(-R+\frac{v_\mathrm{ir}}{2}t\right)\sin(\omega t)-\frac{\sqrt{3}v_\mathrm{ir}}{2}t\cos(\omega t). \end{align*}$$Az (\(\displaystyle x\) , \(\displaystyle y\)) pontok ezután már sokféle programmal könnyen ábrázolhatók (3. ábra).
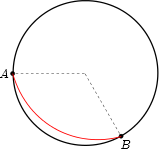
3. ábra
Statistics:
47 students sent a solution. 5 points: Barna Márton, Bencze Mátyás, Csiszár András, Czirják Márton Pál, Fehérvári Donát, Gerendás Roland, Gyenes Károly, Hegedüs Márk, Masa Barnabás, Seprődi Barnabás Bendegúz, Sütő Áron, Szabó Donát, Tóth Hanga Katalin, Zólomy Csanád Zsolt. 4 points: Bernhardt Dávid, Csapó András, Csóka Péter, Erős Fanni, Fajszi Karsa, Monok Péter, Tóth Kolos Barnabás. 3 points: 2 students. 2 points: 12 students. 1 point: 8 students. 0 point: 2 students.
Problems in Physics of KöMaL, February 2024
