KöMaL Problems in Mathematics, December 2013
Please read the rules of the competition.
Show/hide problems of signs:
 |
Problems with sign 'K'Deadline expired on January 10, 2014. |
K. 397. A certain island that is also a country has its own currency, the wooden knut. Everyone pays tax to the state (independently of the size of his or her income). The tax is a certain percentage of the income. However, the government supports the raising of children, therefore every family receives a tax reduction of a certain number of wooden knuts per child (for example, the reduction is twice as many wooden knuts for two children as for a single child). One particular family has an annual income of 1500000 wooden knuts and one child. They pay a tax of 150000 wooden knuts. Another family has an annual income of 2500000 wooden knuts, two children and a tax of 225000 wooden knuts to pay. What percentage of the income is the tax on the island, and what is the tax allowance per child?
(6 pont)
solution (in Hungarian), statistics
K. 398. In the six-digit number 135726, the digit in the thousands' place (the 5) is equal to the double of the number of hundred thousands plus the number of ten thousands (that is, 2.1+3). The digit in the hundreds' place (the 7) is equal to the double of the number of ten thousands plus the number of hundred thousands (that is, 2.3+1). The digit in the tens' place is twice the number of hundred thousands, and the digit in the units' place is twice the number of ten thousands. The number given as an example above is divisible by six. Is it true that all numbers of this property are divisible by six?
(6 pont)
solution (in Hungarian), statistics
K. 399. a) How many numbers A are there for which the least common multiple of 66, 88 and A is 1212?
b) How many numbers B less than 1000 are there for which the greatest common divisor of 66, 303 and B is 33?
(6 pont)
solution (in Hungarian), statistics
K. 400. Steve and Kate are running around the football field, and measuring their times. Steve's stopwatch is set to lap times, that is, whenever he presses the button the watch will store the actual time reading and start the measurement from zero again. Kate's stopwatch is set differently: whenever she presses the button, the watch stores the actual time reading, but it will then continue the measurement without resetting zero. The two of them ran four laps together. Unfortunately, Kate forgot to press the button at the ends of the first and third laps. Thus she only had two readings at the end: 164 seconds and 340 seconds. Based on his own watch, Steve told her that they had run the first lap 5 seconds faster than the mean of the four lap times, and that the mean of the second and third lap times was 4 seconds more than the fourth lap time. How long did they take to cover the fourth lap?
(6 pont)
solution (in Hungarian), statistics
K. 401. In the rectangle ABCD, AB=1 and . A regular triangle is drawn over side AB on the inside, and another regular triangle on side BC is drawn on the outside. The points P and Q are obtained, respectively. What is the area of triangle APQ?
(6 pont)
solution (in Hungarian), statistics
K. 402. Prove that if the reciprocals of two consecutive odd numbers are added, the result is a fraction in which the numerator and denominator are the two smaller members of a Pythagorean triple.
(6 pont)
 |
Problems with sign 'C'Deadline expired on February 10, 2014. |
C. 1196. There are four children in a certain family. Santa brought them some jelly filled candies. The kids re-distributed the candies as follows: Olivia gave half of her candies to Peter. Then Peter generously passed on one third of all candies with him to Rob, and Rob passed on one fourth of his candies to Sarah. Then Sarah observed: ``If I gave Olivia one fifth of my candies, then each of us would have the same number of candies.'' What was the initial distribution of candies? What is the smallest possible number of all candies that the kids have?
(5 pont)
solution (in Hungarian), statistics
C. 1197. Point E lies on side AD of a square ABCD of area 64 units. Point F lies on the extension of side AB beyond vertex B. These two points and vertex C form an isosceles right-angled triangle of area 50 units. What is the area of triangle AFE?
(5 pont)
solution (in Hungarian), statistics
C. 1198. Solve the following equation of two variables: x2+y2+1=xy+x+y.
(5 pont)
solution (in Hungarian), statistics
C. 1199. The accompanying figure shows a sequence of designs made up of floor tiles. The number of dark grey tiles in the designs is 1,6,13,24,37,..., respectively.
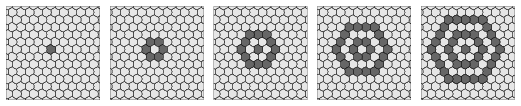
Sophie proved that the number of dark grey tiles in the designs with odd indices in the series is a quadratic function of the index. Determine what number of dark grey tiles there are in the ninety-ninth design according to Sophie's formula.
(5 pont)
solution (in Hungarian), statistics
C. 1200. Solve the equation .
(5 pont)
solution (in Hungarian), statistics
C. 1201. An isosceles triangle AEB is drawn over side AB of a square ABCD on the outside. The measure of its angle, at apex E, is 135o. Let the lines AD and BE intersect at point P, and let CE and AB intersect at Q. Prove that AP=BQ.
(5 pont)
solution (in Hungarian), statistics
C. 1202. The base diameter of a cylindrical tin can is equal to its height. The measures of the surface area in square centimetres and the volume in cubic centimetres are also equal. What is the area of the label that covers the lateral surface of the can entirely?
(5 pont)
 |
Problems with sign 'B'Deadline expired on January 10, 2014. |
B. 4582. Let d(n) denote the number of positive factors of the positive integer n. Determine those numbers n for which d(n3)=5.d(n).
Suggested by M. Di Giovanni, Győr
(3 pont)
solution (in Hungarian), statistics
B. 4583. The points D and E lie on the line segment AB. In the same half plane, a regular triangle is drawn over each of the line segments AD, DB, AE and EB. The third vertices are F, G, H and I, respectively. Prove that if the lines FI and GH are not parallel, then their intersection lies on the line AB.
Suggested by Sz. Miklós, Herceghalom
(3 pont)
solution (in Hungarian), statistics
B. 4584. A line is drawn through each of two opposite vertices of a parallelogram such that each line intersects the extensions of the sides of the parallelogram at two points. Prove that the four points of intersection form a trapezium.
Suggested by G. Moussong, Budapest
(4 pont)
solution (in Hungarian), statistics
B. 4585. Prove that if x1 x2
x2 x3
x3 x4
x4 x5
x5 0, then
0, then . What is the condition for equality?
Suggested by P. Erben and J. Pataki, Budapest
(3 pont)
solution (in Hungarian), statistics
B. 4586. K, L and M are points of sides AB, AC and BC of triangle ABC, respectively. KL is parallel to BC, KL=LC, and . Prove that LM=AK.
(Kvant)
(4 pont)
solution (in Hungarian), statistics
B. 4587. Solve the equation .
(3 pont)
solution (in Hungarian), statistics
B. 4588. The point D lies in the interior of a triangle ABC. Lines CD, AD and BD intersect sides AB, BC and CA at the points E, F and G, respectively. The intersection of lines EG and AF is H, and that of lines EF and BG is I. Show that the lines AB, FG and HI are concurrent.
Suggested by Sz. Miklós, Herceghalom
(5 pont)
solution (in Hungarian), statistics
B. 4589. Is there a natural number n for which the numbers can be divided into 10 sets, such that the sum of the numbers in each set is the same?
(6 pont)
solution (in Hungarian), statistics
B. 4590. Two points and a circle k all lie on the surface of the same sphere. The circle passes through exactly one of the two points. How many circles are there on the sphere that pass through both points and are tangent to the circle k?
(5 pont)
solution (in Hungarian), statistics
B. 4591. Let  be an irrational number. For every positive integer q, let
be an irrational number. For every positive integer q, let , that is, the distance from the closest fraction that can be represented with a denominator of q (not necessarily cancelled to lowest terms). Show that there exists a k such that
.
Suggested by P. Maga, Budapest
(6 pont)
 |
Problems with sign 'A'Deadline expired on January 10, 2014. |
A. 602. Let ABC be a non-equilateral triangle. Consider those equilateral triangles XYZ whose vertices X, Y and Z lie on the lines BC, CA and AB, respectively. Show that the locus of centers of such triangles XYZ is a pair of parallel lines which are perpendicular to the Euler line of the triangle ABC.
Proposed by: András Hraskó, Budapest
(5 pont)
solution (in Hungarian), statistics
A. 603. Let  be a real number. For every positive integer q, let
be a real number. For every positive integer q, let , that is, the distance from the closest fraction that can be represented with a denominator of q (not necessarily cancelled to lowest terms). Show that the sequence
is convergent.
(5 pont)
solution (in Hungarian), statistics
A. 604. There is given a (2n-1)×(2n+1-1) table with integer entries. Prove that it is possible to delete 2n-1 columns from the table in such a way that in each row of the remaining (2n-1)×2n subtable the sum of entries is even.
(5 pont)
Upload your solutions above.
