KöMaL Problems in Mathematics, January 2018
Please read the rules of the competition.
Show/hide problems of signs:
 |
Problems with sign 'K'Deadline expired on February 12, 2018. |
K. 571. The headmaster of a school issued a decree that the legs of students' trousers must not be shorter than one fifth of their height. In the investigation of Sam's trousers length, the ethical committee concluded that the legs of his trousers were shorter than allowed, by exactly \(\displaystyle \frac{2}{7}\) of the allowed minimum length. In addition, they also established that a 3-cm increase of the length of his trousers legs would still make it 20% shorter than allowed. How tall is Sam?
(6 pont)
solution (in Hungarian), statistics
K. 572. Tom Sawyer and Huckleberry Finn were painting the fence together. It would take Tom 3 hours to paint the whole fence alone, and it would take Huck 4 hours to do it alone. However, when they work together, their working speed decreases by 20% since they are doing pranks on each other continually. The two of them started working at noon, but after a while Huck was getting bored, so he decided to go fishing instead. Tom spent 10 minutes trying to persuade him to continue (during that time, neither of them did any painting at all), without success. So he threw a dead rat at Huck, and finished the job alone. He was done at 2:34. When did Huckleberry Finn stop painting?
(6 pont)
solution (in Hungarian), statistics
K. 573. Kate, Alex and Steve went to the sweet shop. Kate bought 9 identical boxes of sweets for Christmas, but she only had \(\displaystyle 11\,000\) forints (Hungarian currency) on her, so she borrowed all the change that Alex had. With that, she just had the right amount of money to pay for the sweets. Then Alex also thought that these sweets would make nice Christmas presents so he decided to buy 13 boxes of the same kind. Since he only had \(\displaystyle 15\,000\) forints left now, he borrowed all the change that Steve had on him. Thus he just had the right amount of money to pay for his sweets. Given that the price of a box of sweets ends in 0 and the amounts borrowed by Kate and by Alex were both less than 1000 forints, how much does Kate owe Alex, and how much does Alex owe Steve?
(6 pont)
solution (in Hungarian), statistics
K. 574. The sum of the digits of a positive number \(\displaystyle N\) is the same as the sum of the digits in its double.
\(\displaystyle a)\) Find a two-digit number, a three-digit number, and a four-digit number with this property.
\(\displaystyle b)\) Show that \(\displaystyle N\) is divisible by 9.
(6 pont)
solution (in Hungarian), statistics
K. 575. Six people are having a meeting. Among any three participants there are two who do not know each other. Prove that there is a set of three participants who do not know each other at all. (Acquaintance is mutual.)
(6 pont)
solution (in Hungarian), statistics
K. 576. A box contains some red and blue balls. If a ball is picked at random, the probability of its being blue is \(\displaystyle \frac{2}{5}\). If one blue ball is removed from the box, the probability of a randomly selected ball being red will be \(\displaystyle \frac{5}{8}\). How many balls are there in the box?
(6 pont)
 |
Problems with sign 'C'Deadline expired on February 12, 2018. |
C. 1455. The currency used on a distant island consists of coins of unusual denominations. The basic units are three different one-digit numbers, and there are their multiples, too: ten times, a hundred times, and also a thousand times their value. The price of one kilo of coconut may be paid with two identical coins plus a third coin of different value. In order to pay for a kilo of passion fruit, which costs twice as much, the third coin needs to be replaced by the coin with 10 times its value. Given that no coin has a denomination of 1 and the largest denomination is 7000, what other coins are used on the island?
(5 pont)
solution (in Hungarian), statistics
C. 1456. Prove that no perfect square can be represented in the form \(\displaystyle 3^a+9^b+1\) (\(\displaystyle a\), \(\displaystyle b\) are positive integers).
(5 pont)
solution (in Hungarian), statistics
C. 1457. An isosceles right-angled triangle inscribed in a circle is rotated through 45 degrees about the centre of the circle. Find the perimeter and area of the intersection of the two triangles.
(5 pont)
solution (in Hungarian), statistics
C. 1458. Solve the following equation on the set of real numbers:
\(\displaystyle \sqrt{x+11} + \sqrt{x^2+11x} -\sqrt{x} -x=4. \)
(5 pont)
solution (in Hungarian), statistics
C. 1459. Reflect the parabola \(\displaystyle y=x^2\) about the point \(\displaystyle F\left(0,\frac 14\right)\). At what angle do the two parabolas intersect?
(5 pont)
solution (in Hungarian), statistics
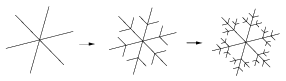
C. 1460. A special snowflake with rotational symmetry is developing as follows: in every second, a new branch of one third the length grows from the midpoint of each terminal branch of the snowflake. (The diagram shows the initial shape of the snowflake and the two successive stages of the process.) Given that the diameter of the snowflake is 4.32 mm, how many terminal branches of length 10 micrometres will it have in 6 seconds?
(5 pont)
solution (in Hungarian), statistics
C. 1461. The operation \(\displaystyle \circ\) is defined on positive integers. Given that \(\displaystyle i)\) \(\displaystyle 1 \circ 1=3\); \(\displaystyle ii)\) \(\displaystyle a\circ b=b\circ a\) for all \(\displaystyle a\), \(\displaystyle b\); \(\displaystyle iii)\) \(\displaystyle a \circ (b+1) = a\circ b + (a+1) + 2b\) for all \(\displaystyle a\), \(\displaystyle b\), determine the value of \(\displaystyle 2017\circ 2018\).
(5 pont)
 |
Problems with sign 'B'Deadline expired on February 12, 2018. |
B. 4921. Let \(\displaystyle n\) and \(\displaystyle k\) denote positive integers. Prove that given \(\displaystyle n+k\) integers it is always possible to select at least \(\displaystyle (k+1)\) numbers out of them such that their sum is divisible by \(\displaystyle n\).
Proposed by Z. Gyenes, Budapest
(5 pont)
solution (in Hungarian), statistics
B. 4922. Find the integer solutions of the following simultaneous equations:
\(\displaystyle 3x-y^{2} =\frac{z}{2},\)
\(\displaystyle 3y+x^{2} =\frac{3z}{2}.\)
Proposed by B. Bíró, Eger
(3 pont)
solution (in Hungarian), statistics
B. 4923. The interior angle bisector drawn from vertex \(\displaystyle A\) of triangle \(\displaystyle ABC\) intersects side \(\displaystyle BC\) at \(\displaystyle E\), and the interior angle bisector drawn from vertex \(\displaystyle B\) intersects side \(\displaystyle AC\) at \(\displaystyle F\). Let \(\displaystyle O\) denote the centre of the inscribed circle of the triangle. What may be the size of the angle at \(\displaystyle C\) if the sum of the areas of \(\displaystyle \triangle OFA\) and \(\displaystyle \triangle OBE\) equals the area of \(\displaystyle \triangle AOB\)?
(3 pont)
solution (in Hungarian), statistics
B. 4924. Consider the perpendicular lines drawn from the centres of the escribed circles of a triangle to the corresponding sides. Prove that the three lines are concurrent.
(4 pont)
solution (in Hungarian), statistics
B. 4925. Show that if the mean of the non-negative real numbers \(\displaystyle a_1,a_2,\dots,a_{2017}\) is 1, then the following inequality holds:
\(\displaystyle \frac{a_1}{a_1^{2018} + a_2 + a_3 + \ldots +a_{2017}} + \frac{a_2}{a_2^{2018} + a_3 + a_4 + \ldots +a_{2017} + a_1} + \ldots + \)
\(\displaystyle + \frac{a_{2017}}{a_{2017}^{2018} + a_1 + a_2 + \ldots +a_{2016}} \le 1.\)
(4 pont)
solution (in Hungarian), statistics
B. 4926. In an acute-angled triangle \(\displaystyle ABC\), the feet of the altitudes drawn from \(\displaystyle B\) and from \(\displaystyle C\) are \(\displaystyle D\) and \(\displaystyle E\), respectively. The reflections of point \(\displaystyle E\) in the lines \(\displaystyle AC\) and \(\displaystyle BC\) are \(\displaystyle S\) and \(\displaystyle T\), respectively. The circle \(\displaystyle CST\), centred at \(\displaystyle O\), intersects line \(\displaystyle AC\) again at point \(\displaystyle X\ne C\). Show that lines \(\displaystyle XO\) and \(\displaystyle DE\) are perpendicular.
(Korean problem)
(5 pont)
solution (in Hungarian), statistics
B. 4927. Let \(\displaystyle A\) and \(\displaystyle B\) be finite sets of vectors, and let \(\displaystyle A+B=\{\mathbf{v}+\mathbf{w} \mid \mathbf{v}\in A\), \(\displaystyle \mathbf{w} \in B\}\). Show that \(\displaystyle |A+B|\ge|A|+|B|-1\).
(5 pont)
solution (in Hungarian), statistics
B. 4928. The trunk of an ever-growing tree forks in two at a height of one foot. In the following, the term branch will refer to a section between two joints, with no further joint along its length. Every branch of the ever-growing tree is straight, and terminates one foot higher than its lower end. The branches starting from the upper end of the branch are considered the children of the branch, also called the siblings of each other. Every branch of the tree has at least two children. If a branch does not have exactly two children then it has a sibling with exactly two children. Siblings always have different numbers of children. If a branch has more than two children then it has a sibling with one fewer children. How many branches start from joints at a height of \(\displaystyle n\) feet?
Proposed by M. E. Gáspár, Budapest
(6 pont)
solution (in Hungarian), statistics
B. 4929. The planes of an ellipse \(\displaystyle \mathcal E\) and a hyperbola \(\displaystyle \mathcal H\) in the space are perpendicular. The foci of \(\displaystyle \mathcal E\) are the endpoints of the real axis of \(\displaystyle \mathcal H\), and the foci of \(\displaystyle \mathcal H\) are the endpoints of the major axis of \(\displaystyle \mathcal E\). Let \(\displaystyle A\) be \(\displaystyle B\) two fixed points on different branches of hyperbola \(\displaystyle \mathcal H\), and let \(\displaystyle P\) be an arbitrary point of the ellipse. Prove that the sum of the distances \(\displaystyle PA\) and \(\displaystyle PB\) is independent of the choice of \(\displaystyle P\).
(6 pont)
 |
Problems with sign 'A'Deadline expired on February 12, 2018. |
A. 713. We say that a sequence \(\displaystyle a_1,a_2,\dots\) is expansive if for all positive integers \(\displaystyle j\), \(\displaystyle i<j\) implies \(\displaystyle |a_i-a_j|\ge \frac1{j}\). Find all positive real numbers \(\displaystyle C\) for which one can find an expansive sequence in the interval \(\displaystyle [0,C]\).
Proposed by: Márk Di Giovanni (Cambridge)
(5 pont)
solution (in Hungarian), statistics
A. 714. Consider \(\displaystyle n\ge 2\) pairwise disjoint disks \(\displaystyle D_1,D_2,\dots,D_n\) on the Euclidean plane. For each \(\displaystyle k=1,2,\dots,n\), denote by \(\displaystyle f_k\) the inversion with respect to the boundary circle of \(\displaystyle D_k\). (Here, \(\displaystyle f_k\) is defined at every point of the plane, except for the center of \(\displaystyle D_k\).) How many fixed points can the transformation \(\displaystyle f_n\circ f_{n-1}\circ\cdots\circ f_1\) have, if it is defined on the largest possible subset of the plane?
(5 pont)
solution (in Hungarian), statistics
A. 715. Let \(\displaystyle a\) and \(\displaystyle b\) be positive integers. We tile a rectangle with dimensions \(\displaystyle a\) and \(\displaystyle b\) using squares whose side-length is a power of \(\displaystyle 2\), i.e. the tiling may include squares of dimensions \(\displaystyle 1\times 1\), \(\displaystyle 2\times 2\), \(\displaystyle 4\times 4\) etc. Denote by \(\displaystyle M\) the minimal number of squares in such a tiling. Numbers \(\displaystyle a\) and \(\displaystyle b\) can be uniquely represented as the sum of distinct powers of \(\displaystyle 2\): \(\displaystyle a=2^{a_1}+\cdots+2^{a_k}\), \(\displaystyle b=2^{b_1}+\cdots +2^{b_\ell}\). Show that
\(\displaystyle M=\sum_{i=1}^k \;\sum_{j=1}^{\ell} 2^{|a_i-b_j|}. \)
(5 pont)
Upload your solutions above.
