KöMaL Problems in Mathematics, February 2018
Please read the rules of the competition.
Show/hide problems of signs:
 |
Problems with sign 'K'Deadline expired on March 12, 2018. |
K. 577. Xavier picked three cards out of a deck of French cards, and placed them on the table in a row. He gave the following information on the cards picked:
– One of the three cards is a king, and immediately to the right of this king there are one or two queens.
– One of the three cards is a queen, and immediately to the left of this queen there are one or two queens.
– One of the three cards is a heart, and immediately to the left of this heart there are one or two spades.
– One of the three cards is a spade, and immediately to the right of this spade there are one or two spades.
What cards may there be on the table, from left to right?
(6 pont)
solution (in Hungarian), statistics
K. 578. The positive integers 1 to \(\displaystyle n\) are written in the fields of the upper row of a \(\displaystyle 2 \times n\) table, in increasing order. The same numbers are written in the lower row, in decreasing order. How many positive integers \(\displaystyle n\) smaller than 50 are there for which every number in the upper row is relatively prime to the number directly below?
(6 pont)
solution (in Hungarian), statistics
K. 579. We form 100 pairs out of 105 girls and 95 boys in a random way. The boy-and-boy pairs shake hands, the girl-and-girl pairs give each other a hug, and the mixed pairs start to dance. Show that the number of handshakes taking place is 5 less than the number of hugs.
(6 pont)
solution (in Hungarian), statistics
K. 580. For what right-angled triangles is it true that \(\displaystyle x> 2(z -y)\), provided \(\displaystyle z> y \ge x\)?
(6 pont)
solution (in Hungarian), statistics
K. 581. Find all four-digit square numbers of the form ABBA.
(6 pont)
solution (in Hungarian), statistics
K. 582. How long may a word be if its letters can be ordered in exactly 180 ways? Give an example of a meaningful English word of this type.
(6 pont)
 |
Problems with sign 'C'Deadline expired on March 12, 2018. |
C. 1462. The first term of an arithmetic sequence is \(\displaystyle a_1=3\), and its common difference is 9. Prove that for every natural number \(\displaystyle k\), the number \(\displaystyle 3\cdot 4^k\) occurs among the terms.
(5 pont)
solution (in Hungarian), statistics
C. 1463. \(\displaystyle M\) is an interior point of a regular triangle \(\displaystyle ABC\). The feet of the perpendiculars dropped from \(\displaystyle M\) onto the sides \(\displaystyle AB\), \(\displaystyle BC\) and \(\displaystyle CA\) are \(\displaystyle H\), \(\displaystyle K\) and \(\displaystyle P\), respectively. Prove that
\(\displaystyle (i)\) \(\displaystyle {|AH|}^2+{|BK|}^2+{|CP|}^2= {|HB|}^2+{|KC|}^2+{|PA|}^2\);
\(\displaystyle (ii)\) \(\displaystyle |AH|+|BK|+|CP|= |HB|+|KC|+|PA|\).
(Mathematical Competitions in Croatia)
(5 pont)
solution (in Hungarian), statistics
C. 1464. We say that a natural number \(\displaystyle B\) can be read out of a larger natural number \(\displaystyle A\), if it is possible to erase some of the digits of \(\displaystyle A\) so that \(\displaystyle B \) is obtained by reading the remaining digits, without changing their order. What is the smallest natural number, such that every three-digit number can be read out of it?
(5 pont)
solution (in Hungarian), statistics
C. 1465. Let \(\displaystyle M\) denote the intersection of the lines \(\displaystyle PS\) and \(\displaystyle RT\) passing through the vertices of a regular triangle \(\displaystyle PQR\) and a square \(\displaystyle QRST\). Show that triangle \(\displaystyle PTM\) is isosceles.
(5 pont)
solution (in Hungarian), statistics
C. 1466. A committee had twelve meetings during the course of a year. At every meeting, there were 10 members of the committee present. Any pair of members were present at most once together. What is the minimum possible number of members on the committee?
(5 pont)
solution (in Hungarian), statistics
C. 1467. Let \(\displaystyle A\) and \(\displaystyle B\) denote the intersection of the circle of radius \(\displaystyle 2r\) centred at \(\displaystyle O\), and the circle of radius \(\displaystyle r+1\) passing through \(\displaystyle O\). How long may \(\displaystyle r\) be if the line segment \(\displaystyle AB\) is the diameter of the smaller circle?
(5 pont)
solution (in Hungarian), statistics
C. 1468. Prove that for all non-negative numbers \(\displaystyle a\) and \(\displaystyle b\)
\(\displaystyle \frac12 {(a+b)}^2+\frac14(a+b)\ge a\sqrt b+b\sqrt a\,. \)
When will the equality hold?
(5 pont)
 |
Problems with sign 'B'Deadline expired on March 12, 2018. |
B. 4930. Every inhabitant of a village belongs to one of three religious faiths: they either worship the Sun God, the Moon God or the Earth God. Regulations of these faiths require that a shrine should have the minimum possible total distance from all the houses of the village (whatever the faith of those living in the houses). Given that the worshippers of the Sun God already have a shrine in the village, and those of the Moon God have one, too, show that the worshippers of the Earth God can also build a shrine for themselves. (The village lies on flat terrain, and the shrines and the houses of the village can be considered pointlike.)
(3 pont)
solution (in Hungarian), statistics
B. 4931. Prove that if \(\displaystyle a\), \(\displaystyle b\), \(\displaystyle c\) are the sides of a triangle then
\(\displaystyle \frac{a^2(b+c)+b^2(a+c)}{abc}>3. \)
(3 pont)
solution (in Hungarian), statistics
B. 4932. The Great Bestiary of Wonderland features a dragon for every week of the year. All dragons have different ages. The youngest dragon, named Aloysius has 13 heads. The second youngest one, Bartholomeus has 14 heads, ... (and so on, each dragon in the order of their ages has one more head than the previous one). The oldest dragon, Zebulon has 64 heads. Wonderland monks are writing the Giant Codex of Dragon Tales. A tale may only be included in the Codex if the total number of heads of all the dragons in the tale is exactly 1001. For every pair of tales, the sets of dragons mentioned in the tales are different. Which of the 13-headed Aloysius and the 14-headed Bartholomeus will appear in more tales when the monks are finished with writing down all possible tales?
(5 pont)
solution (in Hungarian), statistics
B. 4933. Find the area of a regular triangle of maximum perimeter inscribed in a unit square.
(4 pont)
solution (in Hungarian), statistics
B. 4934. For any positive integers \(\displaystyle n\) and \(\displaystyle k\), let \(\displaystyle f(n,k)\) denote the number of unit squares cut in two by a diagonal of an \(\displaystyle n\times k\) lattice rectangle. How many number pairs \(\displaystyle n\), \(\displaystyle k\) are there such that \(\displaystyle n\ge k\), and \(\displaystyle f(n,k)=2018\)?
(4 pont)
solution (in Hungarian), statistics
B. 4935. The given circles \(\displaystyle \omega_1\) and \(\displaystyle \omega_2\) lie inside an angle of vertex \(\displaystyle O\), touching the arms. A ray drawn from point \(\displaystyle O\) intersects circle \(\displaystyle \omega_1\) at points \(\displaystyle A_1\) and \(\displaystyle B_1\), and circle \(\displaystyle \omega_2\) at points \(\displaystyle A_2\) and \(\displaystyle B_2\), such that \(\displaystyle OA_1<OB_1<OA_2<OB_2\) (see the diagram). Circle \(\displaystyle \gamma_1\) touches the circle \(\displaystyle \omega_1\) on the inside, and also touches the tangents drawn to circle \(\displaystyle \omega_2\) from point \(\displaystyle A_1\). Similarly, circle \(\displaystyle \gamma_2\) touches the circle \(\displaystyle \omega_2\) on the inside, and also touches the tangents drawn to circle \(\displaystyle \omega_1\) from point \(\displaystyle B_2\). Prove that the radii of the circles \(\displaystyle \gamma_1\) and \(\displaystyle \gamma_2\) are equal.
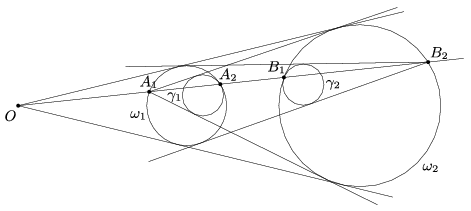
(Kvant)
(5 pont)
solution (in Hungarian), statistics
B. 4936. Let \(\displaystyle AB\) be a fixed chord that is not a diameter in a circle \(\displaystyle k\). The midpoint of \(\displaystyle AB\) is \(\displaystyle F\). Let \(\displaystyle P\) be a point on the circle \(\displaystyle k\), different from \(\displaystyle A\) and \(\displaystyle B\). Let the line \(\displaystyle PF\) intersect circle \(\displaystyle k\) again at \(\displaystyle X\), and let \(\displaystyle Y\) be the reflection of \(\displaystyle X\) in the perpendicular bisector of \(\displaystyle AB\). Prove that there exists a point in the plane that lies on the line \(\displaystyle PY\) for all \(\displaystyle P\).
Proposed by L. Surányi, Budapest
(5 pont)
solution (in Hungarian), statistics
B. 4937. In the plane, a set of lattice quadrilaterals with the following property is selected: however the lattice points are coloured with a finite number of colours, there will always be a selected quadrilateral whose vertices all have the same colour. Prove that there are infinitely many selected lattice quadrilaterals, no two of which have a vertex in common.
Proposed by L. Surányi, Budapest
(6 pont)
solution (in Hungarian), statistics
B. 4938. It is known that it is possible to draw the complete graph with \(\displaystyle 7\) vertices on the surface of a torus (see the Császár polyhedron, for example). 7 points are marked on the side of a mug. We want to connect each pair of points with a curve, so that the curves have no interior points in common. What minimum number of these curves need to lead across the handle of the mug?
(6 pont)
 |
Problems with sign 'A'Deadline expired on March 12, 2018. |
A. 716. Let \(\displaystyle ABC\) be a triangle and let \(\displaystyle D\) be a point in the interior of the triangle which lies on the angle bisector of \(\displaystyle \angle BAC\). Suppose that lines \(\displaystyle BD\) and \(\displaystyle AC\) meet at \(\displaystyle E\), and that lines \(\displaystyle CD\) and \(\displaystyle AB\) meet at \(\displaystyle F\). The circumcircle of \(\displaystyle ABC\) intersects line \(\displaystyle EF\) at points \(\displaystyle P\) and \(\displaystyle Q\). Show that if \(\displaystyle O\) is the circumcenter of \(\displaystyle DPQ\), then \(\displaystyle OD\) is perpendicular to \(\displaystyle BC\).
Proposed by: Michael Ren, Andover, Massachusetts, USA
(5 pont)
solution (in Hungarian), statistics
A. 717. We say that a positive integer is lazy if it has no prime divisor greater than \(\displaystyle 3\). Prove that there are at most two lazy numbers strictly between two consecutive square numbers.
Proposed by: Zoltán Gyenes and Géza Kós, Budapest
(5 pont)
solution (in Hungarian), statistics
A. 718. Let \(\displaystyle \mathbb{R}[x,y]\) denote the set of two-variable polynomials with real coefficients. We say that the pair \(\displaystyle (a,b)\) is a zero of the polynomial \(\displaystyle f\in\mathbb{R}[x,y]\) if \(\displaystyle f(a,b)=0\).
If polynomials \(\displaystyle p,q\in\mathbb{R}[x,y]\) have infinitely many common zeros, does it follow that there exists a non-constant polynomial \(\displaystyle r\in\mathbb{R}[x,y]\) which is a factor of both \(\displaystyle p\) and \(\displaystyle q\)?
(5 pont)
Upload your solutions above.
