KöMaL Problems in Mathematics, September 2020

Problems with sign 'K' Deadline expired on October 12, 2020.

K. 659. How many different quadrilaterals are there whose vertices are selected from the vertices of a regular nonagon so that the quadrilateral contains the centre of the nonagon in its interior? (Congruent quadrilaterals are not considered different.)

(6 pont)

solution (in Hungarian), statistics

K. 660. The squares in the figure were filled in with the numbers 1, 2, 3, 4, 5, 6, 7, 8, 9, and then the sum of the numbers in the two adjacent squares was entered in each circle. Finally, the numbers in a few circles were deleted, and the squares were shaded.

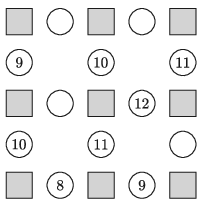
\(\displaystyle a)\) Which numbers are missing from the blank circles?

\(\displaystyle b)\) Enter the original number in each square.

(6 pont)

solution (in Hungarian), statistics

K. 661. The sides of a regular octagon \(\displaystyle ABCDEFGH\) are 2 units long. Two squares, \(\displaystyle BCIM\) and \(\displaystyle GHNP\) are constructed on sides \(\displaystyle BC\) and \(\displaystyle GH\), inside the octagon. Show that the points \(\displaystyle N\) and \(\displaystyle M\) coincide.

(6 pont)

solution (in Hungarian), statistics

K. 662. The first four terms of a sequence are all 1. From the fifth term onwards, each term is obtained by adding the two terms that are four positions and three positions back from that term. How many even numbers are there among the first 150 terms of the sequence?

(6 pont)

solution (in Hungarian), statistics

K. 663. The sum of the squares of three consecutive integers equals the sum of the squares of the following two integers. What may be these five consecutive numbers?

(6 pont)

solution (in Hungarian), statistics

Problems with sign 'C' Deadline expired on October 12, 2020.

C. 1616. Solve the equation

\(\displaystyle x+\frac{1}{y+\frac{1}{\frac{5z}{4}+\frac{13}{6v}}}=\frac{135}{113} \)

where \(\displaystyle x\), \(\displaystyle y\), \(\displaystyle z\), \(\displaystyle v\) are positive integers.

Proposed by B. Bíró, Eger

(5 pont)

solution (in Hungarian), statistics

C. 1617. Is it possible to arrange four \(\displaystyle 2\times 8\) rectangles in the interior of a \(\displaystyle 7\times 15\) rectangle as shown in the figure, without overlapping? (The figure is not to scale.)

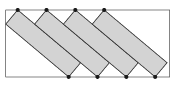
(5 pont)

solution (in Hungarian), statistics

C. 1618. Prove that

\(\displaystyle \frac23\le a_{n+1}-a_n<1 \)

holds for all the terms of the sequence \(\displaystyle a_n=\frac{(n-1)n}{n+1}\), with \(\displaystyle n\ge 1\).

(5 pont)

solution (in Hungarian), statistics

C. 1619. From the midpoint of each side of an acute-angled triangle, drop perpendiculars to the other two sides. Prove that the area of the hexagon formed by the perpendiculars is half the area of the triangle.

(Croatian problem)

(5 pont)

solution (in Hungarian), statistics

C. 1620. Jack Russell fashioned a springboard in his back yard. He carried out measurements to determine that by bending the springboard \(\displaystyle x\) decimetres below horizontal, he could jump to a height of \(\displaystyle 0.5x^2+ax+b\) decimetres. Unfortunately, he forgot the values of \(\displaystyle a\) and \(\displaystyle b\). He only remembers that bending by \(\displaystyle 10\) cm let him jump \(\displaystyle 35\) cm, and a deformation four times as large resulted in a jump four times as high. What may have been Jack's measured values of \(\displaystyle a\) and \(\displaystyle b\)?

(5 pont)

solution (in Hungarian), statistics

C. 1621. The lengths of the sides of a trapezoid circumscribed about a circle are integers that are consecutive terms of an arithmetic progression in some order. Given that the radius of the incircle and the length of the shorter base are both 6, how long are the other three sides?

Proposed by L. Németh, Fonyód

(5 pont)

solution (in Hungarian), statistics

C. 1622. Prove that the area bounded by the graphs of the functions \(\displaystyle y=1-|x-1|\) and \(\displaystyle y=|2x-a|\) is less than \(\displaystyle \frac13\) for \(\displaystyle 1<a<2\).

(Croatian problem)

(5 pont)

solution (in Hungarian), statistics

Problems with sign 'B' Deadline expired on October 12, 2020.

B. 5110. The tangents drawn to the inscribed circle of an isosceles triangle, parallel to the sides, cut off three small triangles. Prove that in the small triangles lying on the base of the large triangle, the heights drawn to the base are equal to the radius of the inscribed circle of the large triangle.

(3 pont)

solution (in Hungarian), statistics

B. 5111. Let \(\displaystyle a\) and \(\displaystyle b\) be real numbers such that \(\displaystyle a + b = 1\) and \(\displaystyle a^2 + b^2 = 2\). Find the value of \(\displaystyle a^8 + b^8\).

Based on the idea of M. Szalai, Szeged

(3 pont)

solution (in Hungarian), statistics

B. 5112. A deck of card consists of \(\displaystyle p\) red cards and \(\displaystyle k\) blue cards. In how many different ways is it possible to select some of the cards so that the number of red cards should be \(\displaystyle n\) more than the number of blue cards?

(4 pont)

solution (in Hungarian), statistics

B. 5113. Let \(\displaystyle a\), \(\displaystyle b\) and \(\displaystyle c\) denote some given, pairwise relatively prime positive integers. Prove that the equation

\(\displaystyle x^a + y^b=z^c \)

has infinitely many solutions \(\displaystyle (x,y,z)\) where \(\displaystyle x\), \(\displaystyle y\) and \(\displaystyle z\) are positive integers.

(5 pont)

solution (in Hungarian), statistics

B. 5114. A unit cube \(\displaystyle ABCDEFGH\) (see the figure) is cut by a plane \(\displaystyle \mathcal{S}\) that intersects the edges \(\displaystyle AB\) and \(\displaystyle AD\) at the points \(\displaystyle P\) and \(\displaystyle Q\) respectively, such that \(\displaystyle AP=AQ=x\) (\(\displaystyle 0<x<1\)). Let the common point of the edge \(\displaystyle BF\) and \(\displaystyle \mathcal{S}\) be \(\displaystyle R\). What is the distance \(\displaystyle BR\) if \(\displaystyle \angle QPR =120^\circ\)?

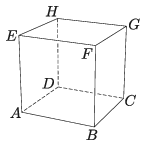
(4 pont)

solution (in Hungarian), statistics

B. 5115. Ali has \(\displaystyle n\) coins in his purse, and Baba has \(\displaystyle n-1\) purses, initially all empty. Baba is playing the following game: he divides the coins (all in the same purse at start) into two purses, with \(\displaystyle a_1\) coins in one of them and \(\displaystyle b_1\) coins in the other (\(\displaystyle a_1,b_1>0\)), and then he writes the product \(\displaystyle a_1b_1\) on a blackboard. Then he continues in the same way: in the \(\displaystyle k\)th move (\(\displaystyle k=2,3,\ldots\)) he selects a purse containing at least two coins, divides them between two empty purses, with \(\displaystyle a_k\) coins in one of them and \(\displaystyle b_k\) in the other (\(\displaystyle a_k,b_k>0\)), and writes the product \(\displaystyle a_kb_k\) on the board.

The game terminates when there is 1 coin in each purse. Then Ali gives as many coins to Baba as the sum of all the products \(\displaystyle a_k b_k\) on the board.

What is the maximum number of coins that Baba may get?

(5 pont)

solution (in Hungarian), statistics

B. 5116. Let \(\displaystyle a,b,c>0\) and \(\displaystyle x,y,z\ge 0\). Prove that if \(\displaystyle x+aby \le a(y+z)\), \(\displaystyle y+bcz \le b(z+x)\), and \(\displaystyle z+cax \le c(x+y)\), then \(\displaystyle x=y=z=0\) or \(\displaystyle a=b=c=1\).

Proposed by G. Stoica, Saint John, Kanada

(6 pont)

solution (in Hungarian), statistics

B. 5117. The points \(\displaystyle A\), \(\displaystyle B\), \(\displaystyle C\), \(\displaystyle D\) (in this order) lie on the same line. On the same side of the line, a regular triangle is drawn on each of the line segments \(\displaystyle AB\), \(\displaystyle BC\) and \(\displaystyle CD\), with the third vertices being \(\displaystyle E\), \(\displaystyle F\), and \(\displaystyle G\), respectively. Let the distances between the adjacent points on the line be \(\displaystyle AB=a\), \(\displaystyle BC=b\), \(\displaystyle CD=c\). Show that the measure of \(\displaystyle \angle EFG\) equals \(\displaystyle 120^{\circ}\) if and only if \(\displaystyle a+c=b\) or \(\displaystyle \frac1a+\frac1c=\frac1b\).

(6 pont)

solution (in Hungarian), statistics

Problems with sign 'A' Deadline expired on October 12, 2020.

A. 780. We colored the \(\displaystyle n^2\) unit squares of an \(\displaystyle n\times n\) square lattice such that in each \(\displaystyle 2\times 2\) part at least two of the four unit squares has the same color. What is the largest number of colors we could have used?

Based on a problem of the Dürer Competition

(7 pont)

statistics

A. 781. We want to construct an isosceles triangle using a compass and a straightedge. We are given two of the following four data: the length of the base of the triangle (\(\displaystyle a\)), the length of the leg of the triangle (\(\displaystyle b\)), the radius of the inscribed circle (\(\displaystyle r\)) and the radius of the circumscribed circle (\(\displaystyle R\)). In which of the six possible cases will we definitely be able to construct the triangle?

Proposed by György Rubóczky, Budapest

(7 pont)

statistics

A. 782. Prove that the edges of a simple planar graph can always be oriented such that the outdegree of all vertices is at most three.

UK competition problem

(7 pont)

statistics