 Problem C. 1152. (January 2013)
Problem C. 1152. (January 2013)
C. 1152. Let and let
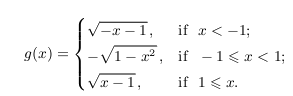
Find the zeros of f-g.
(5 pont)
Deadline expired on February 11, 2013.
Sorry, the solution is available only in Hungarian. Google translation
Megoldás. I.) Ha \(\displaystyle x\geq -3/2\), akkor \(\displaystyle f(x)=x+3/2-3/2=x\). Ekkor 3 eset van.
1.) \(\displaystyle -3/2\leq x<-1\). Ekkor az egyenlet:
\(\displaystyle x=\sqrt{-x-1}.\)
A bal oldal negatív, a jobb oldal nem, ezért nincs megoldás.
2.) \(\displaystyle -1\leq x<1\). Ekkor az egyenlet:
\(\displaystyle x=-\sqrt{1-x^2}.\)
Mivel a jobb oldal legfeljebb 0, ezért \(\displaystyle -1\leq x\leq0\). Négyzetre emelve, majd rendezve:
\(\displaystyle x^2=1-x^2,\)
\(\displaystyle 2x^2-1=0.\)
Ennek megoldása \(\displaystyle \pm\sqrt2/2\), ebből csak az egyik nem pozitív: \(\displaystyle x_1=-\sqrt2/2\).
3.) \(\displaystyle x\geq1\). Az egyenlet: \(\displaystyle x=\sqrt{x-1}\). Négyzetre emelve: \(\displaystyle x^2=x-1\), amiből \(\displaystyle 0=x^2-x+1=(x-1/2)^2+3/4\), ami lehetetlen.
II.) Ha \(\displaystyle x<-3/2\), akkor \(\displaystyle f(x)=-x-3/2-3/2=-x-3\). Ekkor az egyenlet:
\(\displaystyle -x-3=\sqrt{-x-1}.\)
Négyzetre emelve és rendezve:
\(\displaystyle x^2+6x+9=-x-1,\)
\(\displaystyle x^2+7x+10=(x+2)(x+5)=0.\)
A két gyök közül csak az egyik kisebb -3/2-nél: \(\displaystyle x_2=-5\).
Statistics:
240 students sent a solution. 5 points: 119 students. 4 points: 77 students. 3 points: 15 students. 2 points: 6 students. 1 point: 14 students. 0 point: 7 students. Unfair, not evaluated: 2 solutionss.
Problems in Mathematics of KöMaL, January 2013
