Mathematical and Physical Journal
for High Schools
Issued by the MATFUND Foundation
Already signed up?
New to KöMaL?

Problem A. 593. (September 2013)

A. 593. Let a, b, c be positive real numbers. Prove that

\root3\of{7a^2b+1}+\root3\of{7b^2c+1}+\root3\of{7c^2a+1} \le \frac{23}{12}(a+b+c) + \frac1{12} \left(\frac1{a^2}+\frac1{b^2}+\frac1{c^2}\right).

Based on problem 1 of MEMO 2013

(5 pont)

Deadline expired on October 10, 2013.

Solution. The function f(x)=\root3\of{x} is concave on (0,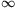\infty), therefore

\root3\of{x} = f(x) \le f(u) + f'(u)(x-u) = \root3\of{u} + \frac1{3\big(\root3\of{u}\big)^2} (x-u)

holds for all u,x>0. In particular, for u=8a3 and x=7a2b+1 we get

\root3\of{7a^2b+1} \le 2a + \frac1{12a^2}(7a^2b+1-8a^3) = \frac43a + \frac7{12}b + \frac1{12a^2}.

Summing up the analogous upper bouds on the other two terms,

\root3\of{7a^2b+1}+\root3\of{7b^2c+1}+\root3\of{7c^2a+1} \le

\le\left(\frac43a + \frac7{12}b + \frac1{12a^2}\right) + \left(\frac43b + \frac7{12}c + \frac1{12b^2}\right) + \left(\frac43c + \frac7{12}a + \frac1{12c^2}\right) =

= \frac{23}{12}(a+b+c) + \frac1{12} \left(\frac1{a^2}+\frac1{b^2}+\frac1{c^2}\right).

Statistics:

39 students sent a solution.
5 points:Ágoston Péter, Balogh Tamás, Barna István, Bereczki Zoltán, Di Giovanni Márk, Fehér Zsombor, Forrás Bence, Ioan Laurentiu Ploscaru, Janzer Barnabás, Maga Balázs, Makk László, Nagy-György Pál, Schwarcz Tamás, Simon 047 Péter, Szabó 789 Barnabás, Szőke Tamás, Tossenberger Tamás, Williams Kada.
4 points:Emri Tamás, Gyulai-Nagy Szuzina, Herczeg József, Kúsz Ágnes, Machó Bónis, Paulovics Zoltán, Petrényi Márk.
3 points:10 students.
2 points:2 students.
0 point:2 students.

Problems in Mathematics of KöMaL, September 2013