 |
Exercises and problems in Physics |
New experimental problem:
m. 205. Measure the coefficient of thermal expansion of wood along the grain and perpendicularly to it. (6 points)
New exercises:
FGy. 3233. A person's heart does approximately a work of 1.6 J during a beat. How much work does it do during a day? How many kg of sand could a machine with such a power output `shovel up' to a height of 1 m? (3 points)
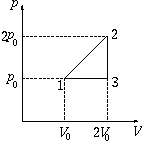FGy. 3234. A certain quantity of gas makes the cycle shown
in the figure. The thermodynamic efficiency of the cycle is
. Can this gas be radon? (4 points)
FGy. 3235. Lying on his stomach at the bottom of a slope that has an inclination of 35o, somebody tries to hit the mark 40 metres upward along the slope with a gun. Where should the gun point if the initial speed of the bullet is 100 m/s and air resistance is neglected? (4 points)
FGy. 3236. A body with a mass of 0.5 kg is fastened to the free end of a light, cylindrical rod with a length of 40 cm and a diameter of 0.5 cm. The period of the small oscillations of the system is measured to be 0.1 second. Determine the value of Young's modulus. (5 points)
FGy. 3237. Electrons inside a solenoid revolve along a circular path. In what direction: according or opposite to that of the electrons moving inside the wire of the solenoid? (3 points)
New problems:
FF. 3238. Two solid, homogeneous spheres of identical
weight and radius start down the same slope at the same time, with no
initial speed. The quality of the surface of each sphere is different:
the coefficient of dynamic friction is 
1=0.1 for one of them
and 2=0.4 for the other one.
a) Which sphere arrives first at the bottom of the slope?
b) Which sphere has a greater kinetic energy at the bottom of the slope? Represent these ratios as a function of the inclination of the slope. (5 points)
FF. 3239. We want to tune the E-string of our guitar to the A-string already tuned, applying the usual method: we hold it down so that it also gives an A, then we touch both strings and listen. We can hear the sound fading every second. By how many percents does the frequency of the false A differ from the required value? Is it higher or lower? The frequency of the tuned A-string is 110 Hz. (4 points)
FF. 3240. Estimate the length of time up to which a fire extinguisher with a volume of 10 litres, containing 4 kg of carbon dioxide can be operated. Assume that the gas leaves the container through a hole with a cross-section of 0.1 cm2, at a uniformly decreasing speed. (5 points)
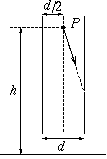FF. 3241. A
particle of charge Q and mass m is released from point
P inside a vertical plane capacitor charged to pd. U, as
shown in the figure. There is a small hole at the point where
the particle reaches the right plate, through which the particle can
pass. What is the trajectory of the particle? In how much time and
where does the particle reach the ground? (Air resistance can be
neglected.) (4 points)
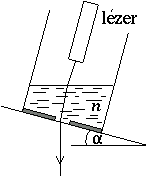FF. 3242. A
recently patented `plummet' consists of a liquid with a suitable
refractive index poured into a cylindrical vessel and of a small
laser. How should the refractive index n be chosen so that the
light refracted by the liquid prism leaves the apparatus practically
vertically when | 
| <10o? How accurate can this apparatus be? (5 points)
New advanced problem:
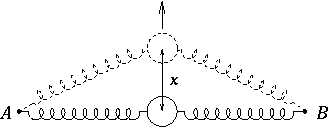FN. 3243. A body of
mass m can move without friction in a horizontal plane. The
springs fixed in points A and B shown in the figure
are initially not expanded, their spring constant is D.
a) What force does the body experience when it is displaced to distance x perpendicularly to the straight line AB? How can this force be approximated for small displacements?
b) What is the period of the oscillations for an amplitude of 2 cm if it is 2 s for an amplitude of 1 cm and AB>>1 cm? (6 points)