KöMaL Problems in Mathematics, February 2010
Please read the rules of the competition.
Show/hide problems of signs:
 |
Problems with sign 'K'Deadline expired on March 10, 2010. |
K. 241. The road from village A to village B is divided into three parts. If the first section were 1.5 times as long and the second one were 2/3 as long as they are now, then the three parts would be all equal in length. What fraction of the total length of the road is the third section?
(6 pont)
solution (in Hungarian), statistics
K. 242. The maximum score on a test is 100 points. The scores of the students are recorded in a computer. When a new score is entered, the program immediately calculates the average of the scores entered so far. While entering the scores of the first five students, the teacher observed that the average increased by 3 points with every score entered. By how many points did the fifth student score more than the first one?
(6 pont)
solution (in Hungarian), statistics
K. 243. Grandma makes muffins with two kinds of filling for her grandchildren: jam and cheese. One time, 40% of the muffins she made were filled with jam. Another time she made 10% more with jam filling and 5% less with cheese filling. By what percentage did the number of all muffins change?
(6 pont)
solution (in Hungarian), statistics
K. 244. Find the largest prime factor of . (11! and 13! denote the products of the whole numbers from 1 to 11, and from 1 to 13, respectively.)
(6 pont)
solution (in Hungarian), statistics
K. 245. Solve the following equations, where x and y denote positive prime numbers.
a) xy(x+y)=2010, b) xy(x+y)=2009.
(6 pont)
solution (in Hungarian), statistics
K. 246. Four different digits are chosen, and all possible positive four-digit numbers of distinct digits are constructed out of them. The sum of the four-digit numbers is 186 648. What may be the four digits used?
(6 pont)
 |
Problems with sign 'C'Deadline expired on April 12, 2010. |
C. 1020. The members of a small group of representatives in the parliament of Neverland take part in the work of four committees. Every member of the group works in two committees, and any two committees have one member in common from the group. How many representatives are there in the group?
(5 pont)
solution (in Hungarian), statistics
C. 1021. P is a point on side AC, and Q is a point on side BC of triangle ABC. The line through P, parallel to BC intersects AB at K, and the line through Q, parallel to AC intersects AB at L. Prove that if PQ is parallel to AB then AK=BL.
(5 pont)
solution (in Hungarian), statistics
C. 1022. A rhombus is constructed out of four metal rods of 20-cm length hinged at the vertices. The longer diagonal is originally 32 cm long. The rhombus is slightly compressed along the longer diagonal. As a result, the other diagonal gets longer by 1.2 as much as the longer one gets shorter. What are the new lengths of the diagonals?
(5 pont)
solution (in Hungarian), statistics
C. 1023. Six cards are numbered 1 to 6 and shuffled. Three cards are drawn in a row, without replacement. What is the probability that the resulting sequence is increasing?
(5 pont)
solution (in Hungarian), statistics
C. 1024. The degree of a polynomial p(x) is at most four. It has zeros and also minima at x1=-3 and x2=5. Given that the polynomial q(x)=p(x-1) is even and has a local maximum value of 256, find the polynomial p(x).
(5 pont)
 |
Problems with sign 'B'Deadline expired on March 10, 2010. |
B. 4242. Is there an n, such that it is possible to walk the 4×n chessboard with a knight touching each field exactly once so that with a last step the knight returns to its original position? What happens if the knight is not required to return to the original position?
(4 pont)
solution (in Hungarian), statistics
B. 4243. Show that 6564+64 is a composite number.
(3 pont)
solution (in Hungarian), statistics
B. 4244. Given the hypotenuse of a right-angled triangle and the radius of the excircle drawn to one of the legs, construct the triangle.
(4 pont)
solution (in Hungarian), statistics
B. 4245. Prove that if a convex polygon is not a parallelogram then it is possible to select three sides, such that the triangle enclosed by the lines of the sides contains
.
(4 pont)
solution (in Hungarian), statistics
B. 4246. Given that the roots x1, x2, x3 of the equation x3-(a+2)x2+(2a+1)x-a=0 satisfy , solve the equation.
(Mathematics Competition for Teacher Training Colleges 1975/2)
(4 pont)
solution (in Hungarian), statistics
B. 4247. Two faces of a cube are ABCD and ABEF. Let M and N denote points on the face diagonals AC and FB, respectively, such that AM=FN. What is the locus of the midpoint of the line segment MN?
(3 pont)
solution (in Hungarian), statistics
B. 4248. Denote the centres of the escribed circles of a triangle ABC by Oa, Ob, Oc, the centre of the incircle by I, and the radius of the circumscribed circle by R. Let A1 be the intersection of the perpendiculars dropped from point Ob onto line AB and from point Oc onto line AC. Show that A1I=2R.
(5 pont)
solution (in Hungarian), statistics
B. 4249. Someone selected n not necessarily different non-negative integers. He wrote down on a sheet of paper all of the (2n-1) sums that can be formed out of the n numbers. Is it possible to determine the original numbers from this information?
(4 pont)
solution (in Hungarian), statistics
B. 4250. The centre of the regular hexagon ABCDEF in the coordinate plane is at the origin O, and vertex A is the point (0,1). Let and
denote the set of points inside or on the boundary of the regular triangles ACE and BDF, respectively. Determine the set of points P for which the vector
can be written in the form
, where
and
.
(4 pont)
solution (in Hungarian), statistics
B. 4251. Let p>3 be a prime number. Determine the last two digits of the number
written in base-p numeral system.
(Based on the idea of G. Mészáros, Kemence)
(5 pont)
 |
Problems with sign 'A'Deadline expired on March 10, 2010. |
A. 500. In space, there are given three prolate spheroids ,
and
(ellipsoids which can be obtained by rotating ellipses about their major axes), and a plane
. The three ellipsoids share one of their foci. Suppose that for each i=1,2,3, the surfaces
and
have exactly two common points with the plane
, and denote by
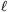 i connecting these common points. Show that the lines
i connecting these common points. Show that the lines 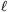 1,
1, 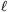 2 and
2 and 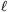 3 are either concurrent or parallel.
3 are either concurrent or parallel.
(Based on the idea of Kristóf Kornis, Budapest)
(5 pont)
solution (in Hungarian), statistics
A. 501. Let p>3 be a prime. Determine the last three digits of
in the base-p numeral system.
(Based on the proposal of Gábor Mészáros, Kemence)
(5 pont)
A. 502. Prove that for arbitrary complex numbers w1,w2,...,wn there exists a positive integer k 2n+1 for which
2n+1 for which .
(5 pont)
Upload your solutions above.
