KöMaL Problems in Mathematics, May 2018
Please read the rules of the competition.
Show/hide problems of signs:
 |
Problems with sign 'C'Deadline expired on June 11, 2018. |
C. 1483. What is the smallest value of the expression \(\displaystyle 6|x - 1| + 5|x - 2| + {4|x - 3|} + 3|x + 4| + 2|x - 5|\)?
(5 pont)
solution (in Hungarian), statistics
C. 1484. The diagonals of a convex quadrilateral \(\displaystyle ABCD\) are not perpendicular. The feet of the perpendiculars dropped from vertices \(\displaystyle A\), \(\displaystyle B\), \(\displaystyle C\), \(\displaystyle D\) onto the sections \(\displaystyle AC\) and \(\displaystyle BD\) are \(\displaystyle A_1\), \(\displaystyle B_1\), \(\displaystyle C_1\), \(\displaystyle D_1\), respectively. (They are different from vertices.) Prove that these points form a quadrilateral similar to the original one.
(5 pont)
solution (in Hungarian), statistics
C. 1485. Let \(\displaystyle x=1^2+3^2+5^2+\cdots+2017^2\) and \(\displaystyle y=2^2+4^2+6^2+\cdots+2018^2\). Evaluate the fraction
\(\displaystyle \frac{y-x}{y+x-(1\cdot 2+2\cdot3+3\cdot4+\cdots+2017\cdot 2018)}. \)
(5 pont)
solution (in Hungarian), statistics
C. 1486. A regular triangle \(\displaystyle ABC\) and a circle \(\displaystyle k\) are both centred at point \(\displaystyle O\), and have an equal area of \(\displaystyle \sqrt{\frac{\pi}{\sqrt{27}}}\). Let the extensions of line segments \(\displaystyle AO\), \(\displaystyle BO\), \(\displaystyle CO\) intersect circle \(\displaystyle k\) at points \(\displaystyle A'\), \(\displaystyle B'\), \(\displaystyle C'\), respectively. Find the exact value of the area of the hexagon \(\displaystyle AC'BA'CB'\).
(5 pont)
solution (in Hungarian), statistics
C. 1487. Nine actors take part of acting exercises each involving three characters. With what minimum number of exercises is it possible to make sure that every pair of actors play together at least once?
(5 pont)
solution (in Hungarian), statistics
C. 1488. Given that any three line segments out of a set of five can be used as sides to construct a (non-degenerate) triangle, prove that at least one of the triangles obtained in this way is acute-angled.
(5 pont)
solution (in Hungarian), statistics
C. 1489. In the lower left corner of a chessboard there is a black bishop, and in the lower right corner there is a white bishop. Each bishop moves up the board in steps of one unit, remaining on fields of its own colour. In each step, it may move to the left or to the right, at random, until it reaches the top row. What is the probability that the black bishop ends up to the right of the white bishop?
(5 pont)
 |
Problems with sign 'B'Deadline expired on June 11, 2018. |
B. 4957. A set with positive integer elements is said to be jolly good if it does not contain a pair of numbers whose difference is 2. How many jolly good subsets does the set \(\displaystyle \{1, 2, 3,\ldots, 10\}\) have?
Proposed by S. Róka, Nyíregyháza
(3 pont)
solution (in Hungarian), statistics
B. 4958. The sides of a triangle are \(\displaystyle a\), \(\displaystyle b\), \(\displaystyle c\), the radius of the inscribed circle is \(\displaystyle r\), and the radius of the circumscribed circle is \(\displaystyle R\). Prove that if
\(\displaystyle a+b+c =\frac{4}{rR} \quad\text{and}\quad \sqrt{ab}+\sqrt{bc}+\sqrt{ca} =6, \)
then \(\displaystyle R=2r\).
(Romanian competition problem)
(4 pont)
solution (in Hungarian), statistics
B. 4959. Barnaby has \(\displaystyle n\) marbles in his pocket. When he performs a somersault, each marble has a probability of \(\displaystyle 0<p<1\) to fall out of his pocket, independently of one another. If at least one marble falls out during a somersault then Barnaby will stop performing somersaults. Otherwise he will continue. Given that the probability of having an even number of marbles in his pocket when he stops doing somersaults is 50%, what may be the value of \(\displaystyle n\)?
(4 pont)
solution (in Hungarian), statistics
B. 4960. Let \(\displaystyle P\) be an interior point of triangle \(\displaystyle ABC\), and let \(\displaystyle A^*\), \(\displaystyle B^*\) és \(\displaystyle C^*\) be arbitrary points of the line segments \(\displaystyle AP\), \(\displaystyle BP\) and \(\displaystyle CP\), respectively. Through point \(\displaystyle A^*\), draw parallels to \(\displaystyle BP\) and \(\displaystyle CP\), which intersect sides \(\displaystyle AB\) and \(\displaystyle AC\) at \(\displaystyle A_1\) and \(\displaystyle A_2\), respectively, as shown in the figure. Similarly, the parallels drawn through point \(\displaystyle B^*\) to \(\displaystyle CP\) and \(\displaystyle AP\) intersect sides \(\displaystyle BC\) and \(\displaystyle AB\) at \(\displaystyle B_1\) and \(\displaystyle B_2\), and finally, the parallels drawn through point \(\displaystyle C^*\) to \(\displaystyle AP\) and \(\displaystyle BP\) intersect sides \(\displaystyle AC\) and \(\displaystyle BC\) at \(\displaystyle C_1\) and \(\displaystyle C_2\), respectively. Show that
\(\displaystyle AC_1 \cdot BA_1 \cdot CB_1 = AB_2\cdot BC_2 \cdot CA_2. \)
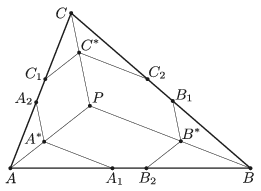
Proposed by J. Kozma, Szeged
(3 pont)
solution (in Hungarian), statistics
B. 4961. The intersection of three unit circles is bounded by the arcs \(\displaystyle \widehat{AB}\), \(\displaystyle \widehat{AC}\) and \(\displaystyle \widehat{BC}\). The perimeter of the intersection is \(\displaystyle K\). Calculate the perimeter of the intersection of the unit circles centred at \(\displaystyle A\), \(\displaystyle B\) and \(\displaystyle C\).
(4 pont)
solution (in Hungarian), statistics
B. 4962. Let \(\displaystyle n\) be a positive integer. Solve the following simultaneous equations on the set of real numbers:
\(\displaystyle a_1^2 + a_1 - 1 = a_2\)
\(\displaystyle a_2^2 + a_2 - 1 = a_3\)
\(\displaystyle \vdots\)
\(\displaystyle a_n^2 + a_n - 1 = a_1.\)
(5 pont)
solution (in Hungarian), statistics
B. 4963. Let \(\displaystyle r_a\) be the radius of the largest escribed circle of a triangle, and let \(\displaystyle R\) denote the radius of the circumscribed circle. Prove that \(\displaystyle r_a \ge \frac32 R\).
A problem from Paul Erdős (1913–1996)
(5 pont)
B. 4964. Is it true that if the functions \(\displaystyle f,g\colon \mathbb{R}\to[0,1]\) are periodic and the function \(\displaystyle f+g\) is also periodic then they have a period in common?
(6 pont)
solution (in Hungarian), statistics
B. 4965. For a vector \(\displaystyle \mathbf x\ne \mathbf 0\), let \(\displaystyle \mathbf e_{\mathbf x}=\frac{\mathbf x} {|\mathbf x|}\). A given plane \(\displaystyle \mathcal{S}\) is parallel, but not identical to the plane of a given (non-degenerate) triangle \(\displaystyle ABC\). Show that there exists a unique \(\displaystyle P\in \mathcal{S}\), such that the vector \(\displaystyle \mathbf e_{\overrightarrow {PA}}+\mathbf e_{\overrightarrow {PB}}+\mathbf e_{\overrightarrow {PC}}\) is perpendicular to \(\displaystyle \mathcal{S}\).
(6 pont)
 |
Problems with sign 'A'Deadline expired on June 11, 2018. |
A. 725. Let \(\displaystyle \mathbb{R}^+\) denote the set of positive real numbers. Find all functions \(\displaystyle f\colon \mathbb{R}^+\to \mathbb{R}^+\) satisfying the following equation for all \(\displaystyle x,y\in\mathbb{R}^+\):
\(\displaystyle f\big(xy+f(y)^2\big) = f(x)f(y)+yf(y). \)
Proposed by: Ashwin Sah, Cambridge, Massachusetts, USA
(5 pont)
A. 726. In triangle \(\displaystyle ABC\) with incenter \(\displaystyle I\), line \(\displaystyle AI\) intersects the circumcircle of \(\displaystyle ABC\) at \(\displaystyle S\ne A\). Let the reflection of \(\displaystyle I\) with respect to \(\displaystyle BC\) be \(\displaystyle J\), and suppose that line \(\displaystyle SJ\) intersects the circumcircle of \(\displaystyle ABC\) for the second time at point \(\displaystyle P\ne S\). Show that \(\displaystyle AI=PI\).
Proposed by: József Mészáros, Galanta, Slovakia
(5 pont)
A. 727. For any finite sequence \(\displaystyle (x_1,\ldots,x_n)\), denote by \(\displaystyle N(x_1,\ldots,x_n)\) the number of ordered index pairs \(\displaystyle (i,j)\) for which \(\displaystyle 1\le i<j\le n\) and \(\displaystyle x_i=x_j\). Let \(\displaystyle p\) be an odd prime, \(\displaystyle 1\le n<p\), and let \(\displaystyle a_1,a_2,\ldots,a_n\) and \(\displaystyle b_1,b_2,\ldots,b_n\) be arbitrary residue classes modulo \(\displaystyle p\). Prove that there exists a permutation \(\displaystyle \pi\) of the indices \(\displaystyle 1,2,\ldots,n\) for which
\(\displaystyle N\big(a_1+b_{\pi(1)},a_2+b_{\pi(2)},\ldots,a_n+b_{\pi(n)}\big) \le \min \big(N(a_1,a_2,\ldots,a_n), N(b_1,b_2,\ldots,b_n) \big). \)
(5 pont)
Upload your solutions above.
